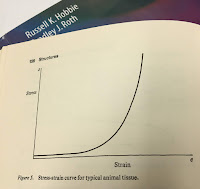
|
| Write Mind, by Eric Maisel. |
Every Saturday morning my wife and I visit the
Rochester Hills Public Library. I like to browse the stacks, and sometimes I find a gem. Recently, I checked out
Write Mind: 299 Things Writers Should Never Say to Themselves (and What They Should Say Instead) by
Eric Maisel.
Write Mind contains some
cognitive therapy jargon that I don’t care for, but its second sentence quotes
Epictetus so it can’t be all bad. Seriously, at its core this delightful book is about attitude. Not all problems can be solved by a positive attitude but some can, whether you are an aspiring writer or a struggling physics student.
Write Mind is aimed at writers. Two hundred and ninety nine times it first states an incorrect, negative attitude (WRONG MIND) and then a better, positive attitude (RIGHT MIND).
To help students of
Intermediate Physics for Medicine and Biology I have paraphrased
Write Mind, providing 29 pairs of statements about physics applied to medicine and biology. Forgive me if sometimes they are corny; I hope you find them useful.
1.
WRONG MIND: Mathematics and medicine are so different; I can’t learn both so I’ll settle for one or the other.
RIGHT MIND: I can master both mathematics and medicine.
2.
WRONG MIND: Physiology requires so much memorization! I will give up and stick to physics.
RIGHT MIND: I can learn both physics and physiology.
3.
WRONG MIND: I hate toy models because they oversimplify biology.
RIGHT MIND: I will gain insight from a toy model, and then analyze its strengths and weaknesses.
4.
WRONG MIND: I have difficulty understanding what some homework problems are asking; I skip those.
RIGHT MIND: Research problems are often ill-defined. I will try my best to understand the question and then answer it.
5.
WRONG MIND: Robert Plonsey, Art Winfree, and John Wikswo have contributed so much; I can never accomplish that much in my career.
RIGHT MIND: I intend to work hard, and take Plonsey, Winfree, and Wikswo as role models.
6.
WRONG MIND: I’m good at math and I love medicine, but I have trouble connecting the two.
RIGHT MIND: Homework problems let me practice connecting math to medicine. Many students struggle with this difficulty. I am not alone.
7.
WRONG MIND:
IPMB and its blog recommend so many books; I don’t have time to read them all, so I won’t read any.
RIGHT MIND: I will find time to read one of the books recommended in
IPMB or its blog. Once I have finished it, I will try to find time for another.
8.
WRONG MIND: I like
IPMB but I don’t have time to do the homework problems.
RIGHT MIND: Today I’ll make time to solve four homework problems. Tomorrow, four more.
9.
WRONG MIND: You have to be a genius to apply physics and mathematics to biology and medicine; I have no chance.
RIGHT MIND: I can learn to apply physics and math to biology and medicine.
10.
WRONG MIND: I am a biologist, and biologists can’t do math.
RIGHT MIND: I intend to learn math.
11.
WRONG MIND: I love math, but my premed advisor says I don’t need math to become a medical doctor.
RIGHT MIND: I choose to learn math and to become a medical doctor.
12.
WRONG MIND: Some students learn the topics in
IPMB easily, but for me they are difficult. I am not meant to understand this subject.
RIGHT MIND: I can understand the topics in
IPMB if I work hard.
13.
WRONG MIND: Applying physics and mathematics to medicine and biology is difficult; I need so many skills. It isn’t worth it. I give up.
RIGHT MIND: I am learning how to apply physics and math to medicine and biology. I’m seeing how it all fits together. It’s so cool!
14.
WRONG MIND: I got my homework back and it was covered with red ink. My instructor is an ass.
RIGHT MIND: I got my homework back and my instructor made many corrections. Such valuable feedback!
15.
WRONG MIND: I spent 30 minutes solving a differential equation. After all that effort, I doubt my solution is correct.
RIGHT MIND: I spent 30 minutes solving a differential equation. Now I will spend 3 minutes plugging my solution back into the differential equation to check that it really works.
16.
WRONG MIND: I solved the differential equation. The solution is complicated and I don’t understand what it means physically.
RIGHT MIND: I solved the differential equation. Now I will examine limiting cases to understand what it means physically.
17.
WRONG MIND: My homework is due Friday. I don’t have to start working on it until Thursday night.
RIGHT MIND: My homework is due Friday. I will start working on it on Monday, leaving time to ask questions if I get stuck.
18.
WRONG MIND: I need to read books by Steven Vogel, Mark Denny, and Knut Schmidt-Nielsen before I am ready to begin my homework.
RIGHT MIND: I would love to read books by Vogel, Denny, and Schmidt-Nielsen, but first I really need to start my homework.
19.
WRONG MIND: I know how to compute an answer to the homework, but it doesn’t mean anything.
RIGHT MIND: The purpose of computation is insight. I will think about my answer until I understand it physically.
20.
WRONG MIND: I have taken a calculus course, but I didn't really master the subject.
IPMB uses calculus a lot; I shouldn’t take a course based on it.
RIGHT MIND: I will take a course based on
IPMB, and use the experience to improve my math skills.
21.
WRONG MIND: Real-world problems are so complicated. The toy models presented in
IPMB won’t prepare me for complex real-world problems.
RIGHT MIND: Solving toy models will help me build the skills and intuition I need to successfully attack more complicated real-world problems.
22.
WRONG
MIND: To solve a homework problem, I search for an equation to put numbers into: “plug-and-chug.”
RIGHT
MIND: I will think before I calculate. After I calculate, I will think if my answer makes sense. I will always think.
23.
WRONG
MIND: Some homework problems ask me to “estimate” something, but they don’t give me all the data I need. What a bunch of BS.
RIGHT
MIND: Part of learning to estimate is to make reasonable assumptions about data I do not have. I will develop this skill.
24.
WRONG
MIND: I derived a complicated equation. I have no idea if it is correct.
RIGHT
MIND: I will check my equation by verifying that it has the correct units. This doesn’t prove it’s right, but it could prove it’s wrong. I will practice this skill.
25.
WRONG
MIND: Why does
IPMB derive so many equations? I don’t need the derivation; I just want to use the equation to calculate numbers.
RIGHT
MIND: A derivation is like a story. The derivation explains what is happening physically, and reminds me what assumptions were made.
26.
WRONG
MIND:
IPMB is always using math to model biological phenomena. This bugs me, and I dislike using “model” as a verb.
RIGHT
MIND: I need to be able to build simple mathematical models of biological phenomena. I must learn to model.
27.
WRONG
MIND: The computed tomography algorithms that create an image from
projections are beautiful. I could never discover something that
profound.
RIGHT MIND: With much hard work, I intend to
discover something new. I will use those beautiful computed tomography
algorithms to motivate me.
28.
WRONG MIND: I received a C- on my first exam, and a D+ on the second. I quit.
RIGHT
MIND: I have learned so much from my mistakes on the first two exams.
Had I gotten A’s on those exams, I wouldn’t be pushing myself hard
enough.
29.
WRONG
MIND: The homework is difficult for me, and my exam average is a C+. I
will never achieve my goal of making new and valuable contributions to
biomedical engineering.
RIGHT MIND: The skills needed
in research are not identical to those needed in the classroom. I have as much to contribute on the job
as the A student.
 |
| WRONG MIND: I would love to write. RIGHT MIND: I intend to write. |