Top Ten Illustrations in Intermediate Physics for Medicine and Biology

I always love top ten lists, so I prepared a list of my top ten illustrations in Intermediate Physics for Medicine and Biology. These are a subjective, personal selections; you may prefer others. I excluded any figure that was reproduced in IPMB from another publication, so many of my favorite images are not listed. Except as noted, Russ Hobbie created these figures, and they appeared first in earlier editions of IPMB on which he was sole author.

10. Figure 15.30. Although this figure is not the most attractive of those in the top ten, I selected it because it is based on Russ’s simulation program MacDose. Be sure to watch Russ’s video based on MacDose; it is a great learning experience.

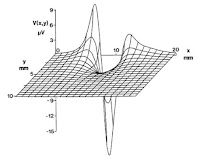
9. Figure 7.13. I helped create this figure when I was in graduate school. Russ asked my PhD advisor John Wikswo if he could supply two figures showing the extracellular potential (Fig. 7.13) and magnetic field (Fig. 8.14) produced by an axon. Wikswo asked me to do the calculations, and he had an illustrator in the lab produce the final drawing.

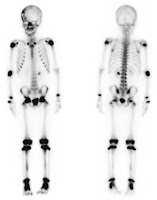
8. Figure 17.19.  This scintillation camera bone scan of a 7-year-old boy is spooky, with ghostly radioactive hot spots. It is one of the many medical images Russ obtained from colleagues at the University of Minnesota. In this case, Bruce Hallelquist provided the photo. IPMB is much the richer for all the images provided by Russ’s friends.

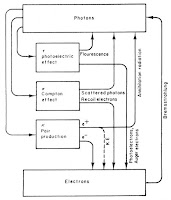
7. Figure 15.15. This figures illustrates the transfer of energy between photons and electrons. I like how it summarizes much of the chapter about the Interaction of Photons and Charged Particles with Matter in a single drawing.

6. Figure 14.24. New in the 4th edition of IPMB, this figure illustrates the blackbody radiation spectrum. It clarifies why the spectrum appears different when plotted versus frequency compared to when plotted versus wavelength.

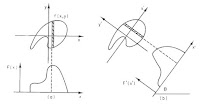
5. Figure 12.12. This illustration defining the projection is critical to understanding tomography. Russ and I liked it so much that we considered using it on the cover of the 4th edition of IPMB, until Springer decided to go with their own cover design that didn’t include a figure from the book.

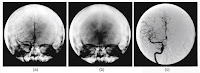
4. Figure 16.23. This image, obtained using digital subtraction angiography, is another medical illustration provided by one of Russ’s colleagues at the University of Minnesota (Richard Geise). I chose it because it is stunningly beautiful.

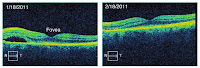
3. Figure 14.16. Color! This optical coherence tomogram of the retina was supplied by Kirk Morgan. A few figures in IPMB go beyond black and white, but this is the only one in glorious full color.

2. Figure 12.6. I like this magnetic resonance image of the brain because it helps build insight into how an image and its Fourier transform are related. It is the first of a series of six images in Chapter 12 prepared by Tuong Huu Le (University of Minnesota, also thanks to Xiaoping Hu) that, by themselves, provide a short course in image processing.

And the winner is….

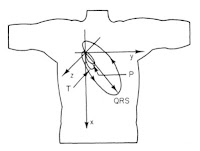
1. Figure 7.16. This picture of the direction of the dipole during the cardiac cycle nicely summarizes the electrocardiogram. My career has focused on the bioelectric behavior of the heart, so it is fitting that my top pick builds on that theme. The reason I chose it, however, is because it was on the cover of the first edition of IPMB, which I used in my first medical physics course taught by John Wikswo at Vanderbilt University.

Enhancement of Human Color Vision by Breaking the Binocular Redundancy

Russ Hobbie and I added a discussion of color vision to the 5th edition of Intermediate Physics for Medicine and Biology.

The eye can detect color because there are three types of cones in the retina, each of which responds to a different wavelength of light (trichromate vision): red, green, and blue, the primary colors. However, the response curve for each type of cone is broad, and there is overlap between them (particularly the green and red cones). The eye responds to yellow light by activating both the red and green cones. Exactly the same response occurs if the eye sees a mixture of red and green light. Thus, we can say that red plus green equals yellow. Similarly, the color cyan corresponds to activation of both the green and blue cones, caused either by a monochromatic beam of cyan light or a mixture of green and blue light. The eye perceives the color magenta when the red and blue cones are activated but the green is not. Interestingly, no single wavelength of light can do this, so there is no such thing as a monochromatic beam of magenta light; it can only be produced my mixing red and blue. Mixing all three colors, red and green and blue, gives white light.

I know that some animals have dichromate vision (only two color receptors), as do some color blind people. Also, a few animals have tetrachromate vision (four color receptors). But I never imagined that I could have enhanced color vision just by wearing a pair of fancy glasses. Could I become a tetrachromat?

Yes! A preprint appeared recently in the biological physics arXiv by Mikhail Kats and his colleagues at the University of Wisconsin about “Enhancement of Human Color Vision by Breaking the Binocular Redundancy” (arXiv:1703.04392). Graduate student and National Science Foundation Graduate Research Fellow Brad Gundlach is the lead author of this fascinating paper. The abstract is given below.

To see color, the human visual system combines the responses of three types of cone cells in the retina—a process that discards a significant amount of spectral information. We present an approach that can enhance human color vision by breaking the inherent redundancy in binocular vision, providing different spectral content to each eye. Using a psychophysical color model and thin-film optimization, we designed a wearable passive multispectral device that uses two distinct transmission filters, one for each eye, to enhance the user’s ability to perceive spectral information. We fabricated and tested a design that “splits” the response of the short-wavelength cone of individuals with typical trichromatic vision, effectively simulating the presence of four distinct cone types between the two eyes (“tetrachromacy”). Users of this device were able to differentiate metamers (distinct spectra that resolve to the same perceived color in typical observers) without apparent adverse effects to vision. The increase in the number of effective cones from the typical three reduces the number of possible metamers that can be encountered, enhancing the ability to discriminate objects based on their emission, reflection, or transmission spectra. This technique represents a significant enhancement of the spectral perception of typical humans, and may have applications ranging from camouflage detection and anti-counterfeiting to art and data visualization.

I’d love to try out a pair of these glasses! I wonder whether they provide merely a subtle change in vision or offer an entirely new visual experience? Also, what would it be like to have each eye receiving different color information? Does the brain need to be trained to handle the additional information, or does it adapt easily? If the enhancement of vision is dramatic, I could easily see these glasses becoming the hot new gadget people clamor for this Christmas. And it all comes from applying physics to medicine and biology.

Five Popular Misconceptions about Osmosis

In Chapter 5 of Intermediate Physics for Medicine and Biology, Russ Hobbie and I discuss osmotic pressure.

5.2 Osmotic Pressure in an Ideal Gas

The selective permeability of a membrane gives rise to some striking effects. The flow of water that occurs because solutes are present that cannot get through the membrane is called osmosis. This phenomenon seems strange when it is first encountered, and explanations are often fraught with misconceptions (Kramer and Myers 2012).

What are these misconceptions that explanations are often fraught with? The reference is to the paper “Five Popular Misconceptions About Osmosis” (American Journal of Physics, Volume 80, Pages 694–699, 2012). The paper raises five questions.

1. Is osmosis limited to mixtures in the liquid state? 
2. Does osmosis require an attractive interaction between solute and solvent? 
3. Can osmosis drive solvent from a compartment of lower to higher solvent concentration? 
4. Can the osmotic pressure be interpreted as the partial pressure of the solute? 
5. What exerts the force that drives solvent across the semipermeable membrane, overcoming both viscous resistance and an opposing hydrostatic pressure gradient?

Later in the paper, the authors answer these questions.

1. The phenomenology of osmosis is the same for gases, liquids, and supercritical fluids. The misconception is that osmosis is limited to liquids. 
2. Osmosis does not depend on an attractive force between solute and solvent. The misconception is that osmosis requires an attractive force. 
3. Osmosis can drive solvent from a lower to a higher solvent concentration compartment. The misconception is that osmosis always happens down a concentration gradient. 
4. The osmotic pressure cannot be interpreted as the partial pressure of the solute. The misconception is that it can. 
5. The semipermeable membrane exerts the force that drives solvent flow. The misconception is that no force is required to explain the flow.

So, how did Russ and I do?

1. We certainly get the first question correct, because our initial explanation is for an ideal gas. 
2. I think we get the second one right too, but it is not as clear, because we restrict our discussion to ideal solutions in which no heat is evolved or absorbed. 
3. We cast our discussion in terms of the chemical potential, and then relate the chemical potential to the hydrostatic pressure and the solute concentration. I don’t think we ever address the issue of solvent concentration. I’ll say we are silent on this one. 
4. We say “Except in an ideal gas, it [the chemical potential] is not the same as the partial pressure (a concept that is not normally used in a liquid).” So we get this one right, and I’m glad we put the not in italics. 
5. In Section 5.9.6 we have a nice discussion about the forces acting on the membrane. But we never really say what force explains the solvent flow. Again, I’ll say we are silent on this one.

Kramer and Myers have an illuminating discussion about the force causing the solvent to cross the membrane (I’ve removed all their references; you can find them in the original paper).

Consider an idealized semipermeable membrane as a force field that repels solute but has no effect on the solvent. The Brownian motion of the solute molecules bring them into occasional contact with this field, at which time they receive some momentum directed away from the membrane. Viscous interactions between solute and solvent then rapidly distribute this momentum to the solvent molecules in the neighborhood of the membrane. In this way, the membrane exerts a repulsive force on the solution as a whole. Since additional pure solvent can freely cross our idealized membrane, it flows into the solution compartment, gradually increasing the hydrostatic pressure in the solution. Thus, a pressure gradient builds up across the thickness of the membrane. This pressure gradient exerts a second force on the solution, capable of counteracting the membrane force. Quantitative treatments show that the pressure difference required to stop solvent flow into a dilute solution is exactly Π = k_BTc_B. Nelson has aptly called the mechanism by which the membrane drives fluid flow the rectification of Brownian motion.

Overall I would say Russ and I do okay. We don’t propagate any of the five misconceptions. We answer three of their questions correctly and are silent on two others. Most of the discussion about osmosis goes back to the 3^rd or earlier editions of IPMB, so Russ is the one who got it right. At least I didn’t screw it up.

My Honors College Class: The Making of the Atomic Bomb

The Making of the Atomic Bomb,
by Richard Rhodes.

This semester I am teaching a class in Oakland University’s Honors College called “The Making of the Atomic Bomb,” based on Richard Rhodes’s book by the same name. The class is a mixture of nuclear physics, a history of the Manhattan Project, and a discussion about World War II (today we discuss Pearl Harbor). I became interested in this topic from the writings of Cameron Reed of Alma College here in Michigan.

The Honors College students are outstanding, but they are from disciplines throughout the university and do not necessarily have strong math and science backgrounds. Therefore the mathematics in this class is minimal, but nevertheless we do a two or three quantitative examples. For instance, Chadwick’s discovery of the neutron in 1932 was based on conclusions drawn from collisions of particles, and relies primarily on conservation of energy and momentum. When we analyze Chadwick’s experiment in my Honors College class, we consider the head-on collision of two particles of mass M₁ and M₂. Before the collision, the incoming particle M₁ has kinetic energy T and the target particle M₂ is at rest. After the collision, M₁ has kinetic energy T₁ and M₂ has kinetic energy T₂.

Intermediate Physics for Medicine and Biology examines an identical situation in Section 15.11 on Charged-Particle Stopping Power.

The maximum possible energy transfer W_max can be calculated using conservation of energy and momentum. For a collision of a projectile of mass M₁ and kinetic energy T with a target particle of mass M₂ which is initially at rest, a nonrelativistic calculation gives

One important skill I teach my Honors College students is how to extract a physical story from a mathematical expression. One way to begin is to introduce some dimensionless parameters. Let t be the ratio of kinetic energy picked up by M₂ after the collision to the incoming kinetic energy T, so t = T₂/T or, using the notation in IPMB, t = W_max/T (the subscript “max” arises because this maximum value of T₂ corresponds to a head-on collision; a glancing blow will result in a smaller T₂). Also, let m be the ratio of M₁ to M₂, so m = M₁/M₂. A little algebra results in the simpler-looking equation

The goal is to unmask the physical behavior hidden in this equation. The best way to proceed is to examine limiting cases. There are three that are of particular interest.

m much less than 1. When m is small (think of a fast-moving proton colliding with a stationary lead nucleus) the denominator is approximately one, so t = 4m. Because m is small, so is t. This means the proton merely bounces back elastically as if striking a brick wall. Little energy is transferred to the lead nucleus.

 m much greater than 1. When m is large (think of a fast-moving lead nucleus smashing into a stationary proton) the denominator is approximately m², so t = 4/m. Because m is large, t is small. This means the lead continues on as if the proton were not even there, with little loss of energy. The proton flies off at a high speed, but because of its small mass it carries off negligible energy.

m equal to 1. When m is one (think of a neutron colliding with a proton, which was the situation examined by Chadwick), the denominator becomes 4, and t = 1. All of the energy of the neutron is transferred to the proton. The neutron stops and the proton flies off at the same speed the neutron flew in.

A mantra I emphasize to my students is that equations are not just things you put numbers into to get other numbers. Equations tell a physical story. Being able to extract this story from an equation is one of the most important abilities a student must learn. Never pass up a chance to reinforce this skill.

Glucose, Mannitol, Sucrose, and Raffinose

The structure of glucose.

You would think by now I would know everything in Introductory Physics for Medicine and Biology; after all, I’m one of the authors. So when thumbing through the book the other day (doesn’t everyone thumb through IPMB when they have a spare moment?) I came across Figure 4.11, showing a log-log plot of the diffusion constant as a function of molecular radius. Four data points stand out—glucose, mannitol, sucrose, and raffinose—because they are plotted as open rather than solid circles. This figure was drawn originally by Russ Hobbie and has appeared in every edition of IPMB. I got to wondering “why did Russ choose to plot those four molecules out of the thousands available?” And then, more specifically, I found myself asking “just what is raffinose anyways?”

To figure all this out, I grabbed the textbook I read in graduate school while auditing the biochemistry class taken by Vanderbilt medical students (Biochemistry, by the late Geoffrey Zubay). These molecules are carbohydrates or, more simply, sugars. Glucose is the canonical example; this six-carbon molecule C₆H₁₂O₆ is “the single most important substrate for energy metabolism” and in humans it is “the single most important sugar in the blood”. It usually exists in a ring conformation. It is a monosaccharide because it consists of a single ring. Other monosaccharides are fructose and galactose, which all have the same formula, C₆H₁₂O₆, but the arrangement of the atoms is slightly different.

Mannitol differs from glucose by having an extra two hydrogen atoms: C₆H₁₄O₆. Technically it’s a sugar alcohol rather than a sugar. You’d think it would act similarly to glucose, but it doesn’t. Mannitol is relatively inert in humans. It doesn’t cross the blood-brain barrier (I discussed the implications of this previously in this blog) and it is not reabsorbed by the kidney like glucose is so it acts as an osmotic diuretic. In Fig. 4.11, the mannitol and glucose data almost overlap, and it is hard to tell which data point is which. According to a paper by Bashkatov et al. (2003), glucose has a larger diffusion coefficient than mannitol, so glucose must be the data point above and to the left, and mannitol below and to the right.

Sucrose is a disaccharide, which means it is two monosaccharides bound together through a “glycosidic linkage”. It’s common table sugar, and consists of a molecule of glucose bound to a molecule of fructose. Russ probably chose to plot sucrose as a typical disaccharide. Two other  disaccharides he could have chosen are lactose (glucose + galactose) and maltose (glucose + glucose).

Raffinose is a trisaccharide, consisting of galactose + glucose + fructose. Therefore, Russ’s choice of plotting glucose, sucrose, and raffinose makes sense: the most important monosaccharide, disaccharide, and trisaccharide. A fun fact about raffinose is that the human digestive tract does not have the enzyme needed to digest it. However, certain gas-producing bacteria in our gut can digest it, resulting in flatulence. You probably won’t be surprised to learn that beans often contain a lot of raffinose.

So, Russ is a clever fellow. He hid a short review of carbohydrate biochemistry in Fig. 4.11. Who knew?