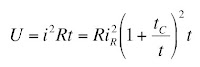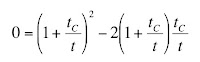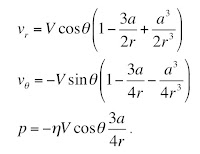Steven Vogel (1940-2015)

Life in Moving Fluids,
by Steven Vogel.

Steven Vogel died on Tuesday. He was the author of several excellent books about the interface between physics and biology. Two that Russ Hobbie and I cite in the first chapter of Intermediate Physics for Medicine and Biology are Vital Circuits (1992) and Life in Moving Fluids (1994), which is one of the books featured in the IPMB Ideal Bookshelf. I posted two blog entries about Vogel’s book Glimpses of Creatures in Their Physical Worlds, here and here. I quote him extensively in a blog entry about the Law of Laplace, in a blog entry about Murray’s law, and in a blog entry about the Reynolds number. His other books I have enjoyed include Life’s Devices, Cats’ Paws and Catapults, and Prime Mover. Reading The Life of a Leaf remains on my to-do list.

I learned the sad news of Vogel’s death from Raghuveer Parthasarathy’s blog The Eighteenth Elephant. There is little I can add to his eloquent tribute. I attended the same conference that Parthasarathy writes about, which is where I met Vogel. He was a delightful and fascinating man. You can listen to him talk about writing scientific papers here, and read his obituary here.

 Steven Vogel talking about writing scientific papers.

https://www.youtube.com/watch?v=eYBMp5sYchA

I leave you with Vogel’s own words, the first two paragraphs of the Preface from the second edition of Life in Moving Fluids. I don’t own the first edition, but I will try to hunt down for you the “first punning sentence” of the first edition Preface that Vogel refers to. I always love a good pun.

About a dozen years ago, calling up a degree of hubris I now find quite inexplicable, I wrote a book about the interface between biology and fluid dynamics. I had never deliberately written a book, and I had never taken a proper course in fluids. But I had learned through teaching—both something about the subject and something about the dearth of material that might provide a useful avenue of approach for biologist and engineer. Each seemed dazzled and dismayed by the complexity of the other’s domain. The book happened in a hurry, in a kind of race against the impending end of a sabbatical semester, and in a kind of mad fit of passion driven by simple realization (and astonishment) that it was actually happening.

The reception of Life in Moving Fluids turned out to surpass my most self-indulgent fantasies—it reached the people I hoped to reach, from ecologist and marine biologist to physical and applied scientists of various persuasions, and it seems to have played a catalytic or instigational role in quite a few instances. Quite clearly the book has been the most important thing of a professional sort that I’ve ever done: certainly that’s true if measured by the frequency with which the first punning sentence of its preface is flung back at me (That my writing has been more important than my research in furthering my area of science suggests that doing hands-on science, which I enjoy, is really just a personal indulgence—quite a curious state of affairs!)

Note added a few hours after the post: Russ has the first edition. He says the first line of the preface is “Fluid flow is not currently in the mainstream of biology, but it has its place.”

The Mystery of the Flawed Homework Problem

When teaching PHY 325 (Biological Physics) this fall, I assigned my students homework from the 5th edition of Intermediate Physics for Medicine and Biology. One problem comes from Section 7.10 about Electrical Stimulation.

Problem 36. If the medium has a constant resistance, find the energy required for stimulation as a function of pulse duration.

The odd thing is, when I looked in the solution manual to review how to solve this problem, it contained answers to parts (a) and (b), and (b) is the most useful part. Where are (a) and (b)? Somehow when preparing the 5th edition, part (b) was left out (it is missing from the 4th edition too). Nevertheless, part (b) ended up in the solution manual (don’t ask me how). This is what Problem 36 should look like:

Problem 36. The longevity of a pacemaker battery is related to the energy required for stimulation.

(a) Find an expression for the energy U expended by a pacemaker to stimulate the heart as a function of the pulse duration t. Use the Lapicque strength-duration curve (Eq. 7.45), and assume the body and electrodes have a constant resistance R. Sketch a plot of energy versus duration.

(b) In general you want to stimulate using the least energy. Determine what duration minimizes the energy expended per pulse.

I don’t usually solve homework problems from the book in this blog, but because the interesting part of this problem was left out of IPMB I don’t think it will hurt in this case. Also, it provides readers with a sneak peak at the solution manual. Remember that Russ Hobbie and I will only send the solution manual to instructors, not students. So if you are teaching from IPMB and want the solution manual, by all means contact us. If you are a student, however, you had better talk to your instructor.

7.36 Issues such as pacemaker battery life are related to the energy required for electrical stimulation. This problem relates the energy to the strength-duration curve, and provides additional insight into the physical significance of the chronaxie.

(a) Let the resistance seen by the electrode due to the medium be R. The power is i²R. Therefore the total energy is

 (b) The duration corresponding to minimum energy is found by setting dU/dt = 0. We get

which reduces to t = t_C. The minimum energy corresponds to a duration equal to the chronaxie.

In the 5th edition’s solution manual, each problem has a brief preamble (in italics) explaining the topic and describing what the student is supposed to learn. We also mark problems that are higher difficulty (*), that complete a derivation from the text (§), and that are new in the fifth edition (¶). Problem 7.36 didn’t fall into any of these categories. We typically outline the solution, but don’t always show all the intermediate steps. I hope we include enough of the solution that the reader or instructor can easily fill in anything missing.

One thing not in the solution manual is the plot of energy, U, versus duration, t. Below I include such a plot. The energy depends on the rheobase current i_R, the chronaxie t_C, and the resistance R.

The energy of a stimulus pulse as a function of pulse duration.

I wonder if this change to Problem 7.36 should go into the IPMB errata? It is not really an error, but more of an omission. After some thought, I have decided to include it, since it was supposed to be there originally. You can find the errata at the book's website: https://sites.google.com/view/hobbieroth. I urge you to download it and mark the corrections in your copy of IPMB.

I hope this blog post has cleared up the mystery behind Problem 7.36. Yet, the curious reader may have one last question: why did I assign a homework problem to my students that is obviously flawed? The truth is, I chose which homework problems to assign by browsing through the solution manual rather than the book (yes, the solution manual is that useful). Problem 7.36 sure looked like a good one based on the solution manual!

Stokes' Flow around a Sphere

When working on the 5th edition of Intermediate Physics for Medicine and Biology, Russ Hobbie and I added a new homework problem about low Reynolds number flow. We ask the reader to analyze the classic example of “Stokes’ flow” or “creeping flow” around a sphere.

Problem 46. Consider a stationary sphere of radius a placed in a fluid of viscosity η moving uniformly with speed V. For low Reynolds number flow, the radial and tangential components of the fluid velocity and the pressure surrounding the sphere are

(a) Show that the no-slip boundary condition is satisfied.

(b) Integrate the shear force and the pressure force over the sphere surface and find an expression for the net drag force on the sphere (Stoke’s law). What fraction of this force arises from pressure drag, and what fraction from viscous drag?

(Everywhere else in our book we correctly write “Stokes’ law” since the law is named after Sir George Stokes, but in this problem we slip up and write “Stoke’s law”. Sorry. I noted this in the errata available on the book website.)

After solving this problem, the reader is probably thinking “this is all well and nice, and I understand now how you get Stokes’ law from the pressure distribution and the viscous drag, but where in the world did you get those weird velocity and pressure distributions?”

First, this example applies to a sphere in water, and water is nearly incompressible. Problem 1.35 shows that incompressibility implies that the velocity u has zero divergence,

The reader should pause now, look up the expression for the divergence in spherical coordinates, and verify that the given velocity really is divergenceless.

Second, the equation describing flow is the Navier-Stokes equation, which is really nothing more than Newton’s second law (F=ma) applied to the fluid. Problem 1.28 provides some insight by deriving a simplified form of the Navier-Stokes equation

 

If we assume a low Reynolds number, we can ignore the two terms on the left-hand side of this equation because they are “inertial” terms arising from the acceleration of the fluid. The two terms on the right-hand side can be generalized to three dimensions, with the pressure term containing the gradient of the pressure and the viscous term containing the Laplacian of the velocity. The resulting Navier-Stokes equation is

To get the expressions given in the new Problem 1.46, solve the Navier-Stokes equation assuming an incompressible fluid. In addition, the boundary conditions are 1) far from the sphere (r much greater than a) the flow is entirely along the z-axis with speed V, and 2) at the sphere surface (r = a) the radial component of the velocity vanishes because the flow is incompressible and the tangential component of the velocity vanishes because of the no-slip boundary condition.

Stokes’ law for the net drag force F, derived in part (b) of Problem 1.46, is F = 6πηaV. Often the drag force is described by a dimensionless coefficient called the drag coefficient, C, equal to F divided by ½ρV²πa². For creeping flow around a sphere, the drag coefficient is

Using the definition of the dimensionless Reynolds number, Re (Eq. 1.62 in IPMB), we find that C = 12/Re. Often the Reynolds number is written in terms of the diameter of the sphere rather than the radius, in which case we get the more commonly quoted relationship C = 24/Re. In many fluid dynamics textbooks you will see C plotted versus Re (usually on log-log graph paper). At low Reynolds number C is inversely proportional to Re as creeping flow predicts. At high Reynolds number the relationship between C and Re is more complex because a turbulent boundary layer forms near the sphere surface. But that’s another story.

The Magnetic Field of a Single Axon (Part 2)

In my last blog entry, I began the story behind “The Magnetic Field of a Single Axon: A Comparison of Theory and Experiment” (Biophysical Journal, Volume 48, Pages 93–109, 1985). I wrote this paper as a graduate student working for John Wikswo at Vanderbilt University. (I use the first person “I” in this blog post because I was usually alone in a windowless basement lab when doing the experiment, but of course Wikswo taught me how to do everything including how to write a scientific paper.) Last week I described how I measured the transmembrane potential of a crayfish axon, and this week I explain how I measured its magnetic field.

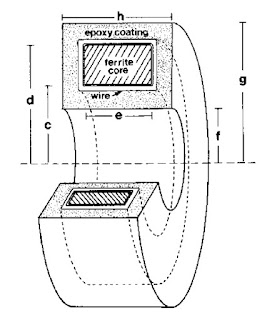A toroid used to measure
the magnetic field of a single axon.

The magnetic field was recorded using a wire-wound toroid (I have talked about winding toroids previously in this blog). Wikswo had obtained several ferrite toroidal cores of various sizes, most a few millimeters in diameter. I wound 50 to 100 turns of 40-gauge magnet wire onto the core using a dissecting microscope and a clever device designed by Wikswo to rotate the core around several axes while holding its location fixed. I had to be careful because a kink in a wire having a diameter of less than 0.1 mm would break it. Many times after successfully winding, say, 30 turns the wire would snap and I would have to start over. After finishing the winding, I would carefully solder the ends of the wire to a coaxial cable and “pot” the whole thing in epoxy. Wikswo—who excels at building widgets of all kinds—had designed Teflon molds to guide the epoxy. I would machine the Teflon to the size we needed using a mill in the student shop. (With all the concerns about liability and lawsuits these days student shops are now uncommon, but I found it enjoyable, educational, and essential.) Next I would carefully place the wire-wound core in the mold with a Teflon tube down its center to prevent the epoxy from sealing the hole in the middle. This entire mold/core/wire/cable would then be placed under vacuum (to prevent bubbles), and filled with epoxy. Once the epoxy hardened and I removed the mold, I had a “toroid”: an instrument for detecting action currents in a nerve. In 1984, this “neuromagnetic current probe” earned Wikswo an IR-100 award. The basics of this measurement are described in Chapter 8 of Intermediate Physics for Medicine and Biology.

In Wikswo’s original experiment to measure the magnetic field of a frog sciatic nerve (the entire nerve; not just a single axon), the toroid signal was recorded using a SQUID magnetometer (see Wikswo, Barach, Freeman, “Magnetic Field of a Nerve Impulse: First Measurements,” Science, Volume 208, Pages 53–55, 1980). By the time I arrived at Vanderbilt, Wikswo and his collaborators had developed a low-noise, low-input impedance amplifier—basically a current-to-voltage converter—that was sensitive enough to record the magnetic signal (Wikswo, Samson, Giffard, “A Low-Noise Low Input Impedance Amplifier for Magnetic Measurements of Nerve Action Currents,” IEEE Trans. Biomed. Eng. Volume 30, Pages 215–221, 1983). Pat Henry, then an instrument specialist in the lab, ran a cottage industry building and improving these amplifiers.

To calibrate the instrument, I threaded the toroid with a single turn of wire connected to a current source that output a square pulse of known amplitude and duration (typically 1 μA and 1 ms). The toroid response was not square because we sensed the rate-of-change of the magnetic field (Faraday’s law), and because of the resistor-inductor time constant of the toroid. Therefore, we had to adjust the signal using “frequency compensation”; integrating the signal until it had the correct square shape.

The amplifier output was recorded by a digital oscilloscope that saved the data to a tape drive. Another of my first jobs at Vanderbilt was to write a computer program that would read the data from the tape and convert it to a format that we could use for signal analysis. We wrote our own signal processing program—called OSCOPE, somewhat analogous to MATLAB—that we used to analyze and plot the data. I spent many hours writing subroutines (in FORTRAN) for OSCOPE so we could calculate the magnetic field from the transmembrane potential, and vice versa.

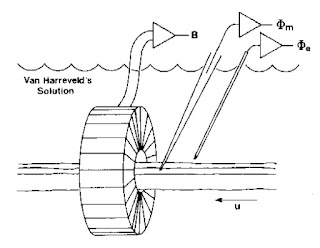An experiment to measure the
transmembrane potential, the extracellular potential,
and the magnetic field of a single axon.

Once all the instrumentation was ready, the experiment itself was straightforward. I would dissect the ventral nerve cord from a crayfish and place it in a plexiglass bath (again, machined in the student shop) filled with saline (or more correctly, a version of saline for the crayfish called van Harreveld’s solution). The nerve was gently threaded through the toroid, a microelectrode was poked into the axon, and an electrode to record the extracellular potential was placed nearby. I would then stimulate the end of the nerve. It was easy to excite just a single axon; the nerve cord split to go around the esophagus, so I could place the stimulating electrode there and stimulate either the left or right half. In addition, the threshold of the giant axon was lower than that of the many small axons, so I could adjust the stimulator strength to get just one giant axon.

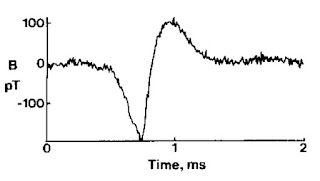The magnetic field of a single axon.

When I first started doing these experiments, I had a horrible time stimulating the nerve. I assumed I was either crushing or stretching it during the dissection, or there was something wrong with the saline solution, or the epoxy was toxic. But after weeks of checking every possible problem, I discovered that the coaxial cable leading to the stimulating electrode was broken! The experiment had been ready to go all along; I just wasn’t stimulating the nerve. Frankly, I now believe it was a blessing to have a stupid little problem early in the experiment that forced me to check every step of the process, eliminating many potential sources of trouble and giving me a deeper understanding of all the details. 

As you can tell, a lot of effort went into this experiment. Many things could, and did, go wrong. But the work was successful in the end, and the paper describing it remains one of my favorites. I learned much doing this experiment, but probably the most important thing I learned was perseverance.