One Hot Summer: Dickens, Darwin, Disraeli, and the Great Stink of 1858

One Hot Summer,
by Rosemary Ashton.

I recently finished Rosemary Ashton’s book One Hot Summer: Dickens, Darwin, Disraeli, and the Great Stink of 1858. Her prologue begins

What was it like to live in London through one of the hottest summers on record, with the Thames emitting a sickening smell as a result of the sewage of over two million inhabitants being discharged into the river? How did people cope with the extraordinary heat leading up to the hottest recorded day, Wednesday, 16 June 1858? What did those living or working near the Thames—including at the Houses of Parliament and the law courts in Westminster Hall—do when they found their circumstances intolerable? What did the newspapers say?

Ashton proposes to examine London for just a few months in the summer of 1858, providing a snapshot of one moment in Victorian England. Such a microhistory provides insight into the life of mid-19th century Britain.

Microhistory, the study in depth and detail of historical phenomena, can uncover hitherto hidden connections, patterns, and structures. Some events and incidents are revealed over time to have been life changing or nation building. Examples from 1858 are the tackling of London’s sewage and the resultant improvement of public health, Brunel’s engineering feats, the initial laying of the Atlantic telegraph cable, the beginnings of a long process of attaining justice and equality in the matter of marriage and divorce, and the transformation of the miscellaneous medical practice into a proper profession.

She focuses on the novelist Charles Dickens, biologist Charles Darwin, and politician Benjamin Disraeli.

A comparatively neglected time in Disraeli’s career can be shown to have been remarkably important in bringing him to prominence. The attention of historians and biographers has focused hitherto on his reckless youth, his racy novels, his controversial journalism, and his late-won success from 1868, when he finally became prime minister. His hard work in the parliamentary session of 1858, particularly in the hectic weeks before the summer break beginning on 2 August, and his success in turning round a hostile press and distrustful colleagues by his efforts, deserve to be acknowledged. In Dickens’s case his painful and self-exposing actions in connection with his failed marriage have been fully discussed, but no detailed account exists of the day-to-day struggles he faced in the long summer which followed his catastrophic error of judgment in advertising his separation from his wife in the early days of June. As for Darwin, though much has been written about his abrupt shock and change of plans on receiving in mid-June Wallace’s letter outlining natural selection, little attention has been paid to the interaction between his family life and scientific work in summer 1858.

This idea of a microhistory sounds fun, and I thought readers of Intermediate Physics for Medicine and Biology might be interested in learning about events in the summer of 1858 that influenced physics, biology, and medicine. So, in this blog post I augment Ashton’s analysis by adding incidents from the world of science.

Charles Darwin (age 48, all ages are as of summer 1858) had been developing his theory of evolution by natural selection for twenty years, since returning to England in 1836 after his famous voyage on the HMS Beagle. Over the years he had told his friends Joseph Hooker (age 41) and Charles Lyell (age 61) about his ideas, but had never published them. Ashton describes how on June 18, 1858 Darwin received a letter from Alfred Russel Wallace (age 35), containing a draft of a paper describing the same idea of natural selection as the mechanism of biological evolution, written while Wallace was collecting biological specimens in the Malay Archipelago. Hooker and Lyell arranged to have some early private writings of Darwin’s, along with the paper by Wallace, published on July 1 at a meeting of the Linnean Society of London. 

On the Origin of Species,
by Charles Darwin.

The following year, Darwin published his much more detailed book On the Origin of Species, changing biology forever. One of the most pugnacious of the advocates for natural selection was his young friend Thomas Henry Huxley (age 33), known as “Darwin’s Bulldog.” In 1858 Huxley was the Fullerian Professor of Physiology at London's Royal Institution, and on June 17, 1858 he gave the Royal Society’s annual Croonian Lecture. Darwin’s friend Charles Lyell—winner of the Royal Society’s prestigious Copley Medal in 1858 for his contributions to geology—never completely embraced natural selection.

On June 10, 1858 the botanist Robert Brown died in London, at age 84. In Chapter 4 of IPMB, Russ Hobbie and I write

This movement of microscopic-sized particles, resulting from bombardment by much smaller invisible atoms, was first observed by the English botanist Robert Brown in 1827 and is called Brownian motion.

Brown’s death had an interesting impact on the Darwin/Wallace publications. Ashton writes

By a stroke of luck the death of the former president Robert Brown had induced the [Linnean] society to postpone its summer meeting from 17 June, the day before Darwin received Wallace’s letter, to Thursday, 1 July. This meant that Darwin (and Wallace) would not have to wait until September to have their papers made public.

One of the most famous scientists in England during 1858 was Michael Faraday (age 65). In Chapter 8 of IPMB, Russ and I discuss electromagnetic induction, which underlies transcranial magnetic stimulation of the brain.

In 1831 Michael Faraday discovered that a changing magnetic field causes an electric current to flow in a circuit.

Faraday, Maxwell, and the
Electromagnetic Field,
by Forbes and Mahon.

After a long career at the Royal Institution, Faraday moved from his home at the RI to a house at Hampton Court in 1858. In their book Faraday, Maxwell, and the Electromagnetic Field, Nancy Forbes and Basil Mahon write

As Faraday’s health and mental faculties declined, he began to relinquish his various responsibilities at the Royal Institution, finally handing over the directorship to John Tyndall in 1865. The consequent loss of income, and of his flat, would have been a worry, but in 1858 Prince Albert, a great admirer, had asked the queen to put a house at Hampton Court at his disposal. Faraday had refused at first, fearing the high cost of repairs, but the queen said she would pay. He and Sarah [his wife] moved in, and the new house became his last home.

Although his research career was winding down, Faraday was still a great science communicator. On June 12, 1858 he gave a RI lecture “On the relation of gold to light,” about light scattering from gold colloids (nowadays we would call them gold nanoparticles). He was also famous for his Christmas lectures, which he gave annually throughout the 1850s.

Faraday’s work in electricity and magnetism was carried on by the young James Maxwell (age 27), who was married on June 2, 1858 in Aberdeen, Scotland. That year, Maxwell published his paper “On Faraday’s Lines of Force” (although it had been read before the Cambridge Philosophical Society in late 1855 and early 1856). Forbes and Mahon write

In February 1857, [Maxwell] decided to send a copy of his paper “On Faraday’s Lines of Force” to the great man [Faraday]. No doubt, he did so with some trepidation… He needn’t have worried. As we’ve seen, Faraday’s response was grateful, gracious, and charming. The two had at once formed a rare bond.

In the 1860s Maxwell continued his research on electromagnetism, and eventually developed the four Maxwell’s equations that rival Darwin’s theory of evolution as the most significant scientific contribution of the 19th century.

A Thread Across the Ocean,
by John Steele Gordon.

Besides Faraday and Maxwell, a third great Victorian physicist was William Thomson (age 34), who was one of the main scientists involved in developing the transatlantic telegraph. As part of that effort, in February of 1858 Thomson patented the mirror galvanometer, which is an instrument to measure electrical current. In his book A Thread Across the Ocean, John Steele Gordon describes this device.

In a long submarine cable, immersed in a conducting medium—saltwater—the current if often very low, sometimes no more than ten mircoamperes. (The current in a standard incandescent lightbulb is about 100,000 times as great.) The standard galvanometers then available were often inadequate to detect a signal coming through a cable that would be two thousand miles long. So Thomson—half Einstein, half Edison—developed a much better one. He took a very small magnet and attached a tiny mirror to it. Both together weighed no more than a grain. He suspended the magnet from a silk thread and set it in the middle of the coil of very thin insulated copper wire.

When the faint current flowing through the cable was allowed to flow through the copper coil, it created a magnetic field. This caused the magnet, with its attached mirror, to deflect. Thomson simply directed a beam of light from a shaded lamp onto the mirror and allowed it reflection to hit a graduated scale.

In June of 1858 two ships—the Agamemnon and the Niagara—attempted to meet in the middle of the Atlantic Ocean, splice together the two halves of the cable, and then each pay out the cable as they sailed toward shore: the Niagara toward Newfoundland and the Agamemnon toward Ireland. However, a terrible storm struck the North Atlantic that month, nearly capsizing the Agamemnon with Thomson on board and aborting the mission.

On Sunday, June 20, the storm unleashed a fury such as few sailors ever see and even fewer live to tell about. The caption feared that the coil on the deck, working against its restraints, might break lose and smash through the side, undoubtedly causing the ship to founder.

A second try several weeks later proved more successful. On August 16, the first transatlantic telegraph message was sent between Queen Victoria in England and President James Buchanan in the United States. Unfortunately, the cable soon failed, and it was not until some years later that reliable telegraph service was established across the Atlantic.

Based on his basic research discoveries and his contributions to the telegraph, Thomson became a scientific hero. Gordon writes

In 1892, William Thomson became the first British scientist to be raised to the peerage, when Queen Victoria created him Lord Kelvin of Largs. He has been known ever since as Lord Kelvin. In 1908, the year after he died, the Kelvin temperature scale, devised by him in the 1850s, was named in his honor.

The absolute temperature scale, with Kelvin’s name attached to the unit of temperature, appears throughout IPMB.

Still another notable Victorian physicist was George Stokes (age 36), who at that time was the Lucasian Professor at Cambridge University (a position held earlier by Isaac Newton and later by Stephen Hawking). IPMB often uses Stokes’ law for the viscous force of a small sphere in a fluid. Stokes and Thomson were close friends, and their many letters are preserved. I provide a few excerpts from these letters during late 1857 and 1858.

2 College, Glasgow

Dec. 23, 1857

My Dear Stokes

That principle, in the hydrodynamics of a “perfect liquid”, which I first learned from you, is something that I have always valued as one of the great things of science, simple as it is, and I now see more than ever its importance. One conclusion from it is that instability, or a tendency to run to eddies, or any kind of dissipation of energy, is impossible in a perfect liquid (a fluid with neither viscosity nor compressibility)... [several pages follow with many equations]...Some of the simplest applications of the theory are very interesting: for instance the... case of a circular disc or oblate spheroid, moving... in a perfect [liquid]...

As to Faraday’s magneto-optic experiment, I think my argument that it must depend on a peculiar state of motion induced by magnetic influence (Proceedings R. S. June or July 1856) is unanswerable. Have you considered it?...

It seems like old times for me to be writing you so long a letter, and I am afraid you will be less disposed to be so bored. Your redress simply be not to read it.

With best wishes for a “Merry Christmas” of which there can be no doubt now, I remain

Yours always truly

William Thomson

Stokes responded,

69 Albert Street Regent's Park London N.W.

Feb. 12, 1858

My Dear Thomson,

I have been so very busy of late that your letter has remained for a long time unanswered. I now set to answer it, though I have still got plenty of work before me...

Without having a decided opinion either way I have always inclined to the belief that the motion of a perfect incompressible liquid, primitively at rest, about a solid which continually progressed, was unstable... [pages of math...]

In speculating a good while ago (in fact no great time after Faraday’s discovery) as to the cause of magnetic rotation I naturally tried rotations of the luminiferous ether as suggested by Ampere’s theory...

Yours very truly

G. G. Stokes

Finally, late in 1858, Stokes wrote

The Athenaeum

Oct 5/58

My Dear Thomson,

... It is a great pity to see the [transatlantic] cable in its present state after apparently so successful a laying down. Still the thing has been done and even if this should be utterly lost the matter will not I presume rest there.

I did not go to Leeds this meeting [The British Science Association met in Leeds in 1858]. On the morning of the 27th my wife was safely delivered of a fine boy. She is going on very well but I am afraid her complete recovery will be slow.

Yours very truly

G. G. Stokes

James Joule (age 39) was yet another English physicist of the Victorian era. His name appears repeatedly in IPMB because the unit of energy is named after him. In the 1840s Joule had done pioneering work on the mechanical equivalent of heat and the conservation of energy, and in the 1850s had collaborated to explain the Joule-Thomson effect. In 1858 he was in a train wreck while traveling home from London. Although unhurt, the accident made him reluctant to travel, somewhat isolating him from the scientific community.

Gray’s Anatomy.

 A major event in medicine occurred during the summer of 1858: the publication of the first edition of Gray’s Anatomy. In his article “Happy Birthday, Gray’s Anatomy,” Adrian Flatt (Proc. Bayl. Med. Cent., 22:342–345, 2009) writes

Anatomy Descriptive and Applied was first published in London in the summer of 1858 by two young demonstrators of anatomy in St. George’s Hospital at Hyde Park Corner… These two young men were very different. Henry Gray [age 31] wrote the text; he was 4 years older than Henry Vandyke Carter [age 27], who drew all the illustrations…

The print number of 2000 books had been decided, page size was fixed, and all the paper purchased. Considerable adjustments were successfully made and by mid May 1857, the work was going well but was to be interrupted by the absence of Gray. He had received an invitation to “attend” the Duke of Sutherland on his private yacht sailing around England and Scotland and at the estate at Dunrobin Castle for the next 6 months, from June to November 1857. This was manna from heaven for Gray; service for such an aristocrat would be of enormous help to his practice. Carter continued work on the book, of which the final proof corrections were done in late June or early July 1858, in time for the book to be available for students arriving in September.

Gray died at age 34, just three years after publication of his textbook, of smallpox. Apparently the relationship between Gray and Carter was strained. Flatt states that

Gray never gave Carter one penny from all the royalties the early editions of the book earned.

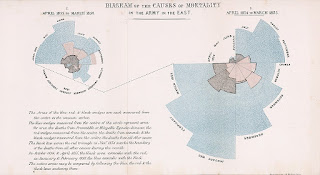Diagram of the causes of mortality
in the army in the East (1858).

Another leading figure of Victorian health care was Florence Nightingale (age 38), the founder of modern nursing. In 1858 Nightingale published Notes on Matters Affecting the Health, Efficiency, and Hospital Administration of the British Army. Founded Chiefly on the Experience of the Late War. Presented by Request to the Secretary of State for War. This work contained a color statistical illustration called “Diagram of the Causes of Mortality in the Army of the East” that showed that epidemic disease—which caused more British deaths during the Crimean War than battlefield wounds—could be controlled by nutrition, ventilation, and shelter. The infographic became known as Nightingale’s “coxcomb.” Her achievements in statistics were so remarkable that in 1858 she was selected as the first woman fellow of the Royal Statistical Society. Two years later she established her nursing school at Saint Thomas’ Hospital in London.

Another noteworthy happening in medicine was the death of John Snow (age 45) on June 16, 1858 (London’s hottest day of that steamy summer). Snow was best known for figuring out the source of the Broad Street cholera outbreak in 1854, when he demonstrated that cholera was being spread through contaminated water from one specific pump. He also studied using ether as an anesthesia during surgery. 

The Ghost Map,
by Steven Johnson.

In his fascinating book The Ghost Map, Steven Johnson writes about the prevailing belief that miasma (bad air) caused disease.

In June 1858, a relentless early-summer heat wave produced a stench of epic proportions along the banks of the polluted Thames. The press quickly dubbed it the “Great Stink”... [Yet] the rates of death from epidemic disease proved to be entirely normal. Somehow the most notorious cloud of miasmatic air in the history of London had failed to produce even the slightest uptick in disease mortality... It's easy to imagine John Snow taking great delight in [this] puzzling data... But he never got the opportunity. He had suffered a stroke in his office on June 10... and died six days later, just as the Great Stink was reaching its peak above the foul waters of the Thames.

Joseph Lister (age 31) was in Edinburgh in 1858, studying the coagulation of blood and inflammation. In the 1860s he developed antiseptic surgery, and later relocated to London. In their article “Joseph Lister: Father of Modern Surgery” (Can. J. Surg., 55:E8–E9, 2012), Dennis Pitt and Jean-Michel Aubin claim that 

it was Lister’s application of germ theory to the care of surgical patients that laid the foundation for what surgeons do now. He directed the minds of physicians and surgeons to the vital necessity of keeping wounds clean and free of contamination.

Finally, in 1858 Elizabeth Garrett Anderson (age 22) was a young woman dreaming of making a career in medicine. She eventually became the first female doctor in the United Kingdom.

Ashton believes that microhistory provides valuable insight into Victorian England. Near the end of her Prologue she concludes

Intense scrutiny of the lives of these men [Dickens, Darwin, and Disraeli, plus Brown, Faraday, Maxwell, Thomson, Stokes, Joule, Gray, Nightingale, Snow, and others] over a short period of a few months allows us to make fresh threads of connection between each of them and the larger society in which they lived, all at a time of public events which provided to be of lasting national importance.

Louis Pasteur, Biological Physicist

Louis Pasteur (1822–1895)

One recurring theme in this blog is how scientists make the transition from working in the physical sciences to studying the biological sciences. Indeed, this theme is intimately related to Intermediate Physics for Medicine and Biology. Recently, I decided to consider a case study of how a prominent scientist straddled physics, biology, and medicine. So, I searched for someone famous who illustrates how one trained in physics can end up contributing to the life sciences. I selected Louis Pasteur.

Louis Pasteur, by Patrice Debré.

I base this study on the biography Louis Pasteur by Patrice Debré (translated from French to English by Elborg Forster). As I read this book, I focused on the key events in Pasteur’s education and early research when he made this transition. 

Pasteur began his career as a physical scientist studying at the École normale supérieure in Paris.

For his doctorate, Pasteur had to submit two theses, one in physics and one in chemistry. The physics thesis brought together several studies concerning the use of the polarimeter… Pasteur’s first studies showed, or rather confirmed, that two isomorphic substances rotate polarized light to the same degree.

Polarization was a new topic in physics at that time. Étienne-Louis Malus, a fellow Frenchman, discovered the Law of Malus, governing how much light passes through two polarizers, in 1808, just 14 years before Pasteur’s birth. Pasteur’s friend and mentor Jean-Baptiste Biot first showed that polarized light could be rotated when passed through certain crystals. Pasteur’s contribution was to prove that crystals formed from tartaric acid could rotate polarized light either clockwise or counterclockwise, depending on the chirality of the crystal (this acid is asymmetric, having two forms that are mirror images of each other, like the left hand and the right hand). In a famous experiment, he inspected the structure of each crystal under a microscope and determined if it was left or right handed. When he then separated the two types of crystals he could obtain rotation in either direction, although a mixture of the two crystals did not rotate light. This discovery, made in 1848, at first appears to arise from physics and chemistry alone, but its relation to biology is that most biological molecules exist in only one version. Handedness matters in biology. Debré writes

In discovering the principles of molecular asymmetry, Pasteur had done nothing less than to forge a key—and this key has unlocked the door to the whole of modern biology… When Pasteur considered asymmetry on a cosmic scale, he went beyond the confines of physics and chemistry to confront the fundamental questions about life.

Pasteur’s next step toward biology came when he was a young professor at the University of Lille.

At the beginning of the academic year 1856, an industrialist of Lille, M. Bigo, whose son Emile was taking Pasteur’s course at the Faculty of Sciences, came to see him. Many manufacturers of beet root alcohol, he said, were having problems with their production…

This led to Pasteur’s research on fermentation, when a microorganism such as yeast brings about a change to a food or beverage, such as producing alcohol. Fermentation and light polarization do not appear to have much in common, but they do.

The findings Pasteur presented to the Academy of Sciences of Lille, and subsequently that of Paris, seemed very different from the studies he had undertaken previously. He was known as a specialist on crystals, and now he had become a theoretician of fermentation. Ranging from polarized planes of light to culture media, his reagents had little in common. Yet the preoccupations that guided Pasteur’s thinking at that period were not really different from those that had haunted him for a long time: he wanted to understand the relationship between life and molecular asymmetry.

The idea that a living microscopic organism was responsible for fermentation was one of Pasteur’s key insights. In fact, there were two types of yeast involved in beet root fermentation. The desirable one produced alcohol. The undesirable one, that led to all the problems, produced lactic acid. Debré concludes

A few years after the request of industrialist Bigo, Pasteur had thus established beyond a doubt that the lactic acid in the vats in the rue d’Esquermes came from an unfortunate contamination with this yeast. He even suggested the means to get rid of this contamination… Pasteur’s research on fermentation created microbiology.

Pasteur’s work on fermentation led to the related question of spontaneous generation. Many scientists at the time thought that living organisms could spontaneously arise in dead and decaying tissue, but Pasteur showed that such decay was always due to germs that entered the tissue from the air.

Pasteur’s transition to biology became complete after Jean-Baptiste Dumas asked him to investigate a disease that was destroying the silkworm industry in France. To address this issue, he needed to learn more biology.

Pasteur came from crystals. Owing to his scant knowledge of animal biology, he was somewhat apprehensive about experiments on animals. As soon as he accepted Dumas’s assignment, he therefore went, along with his assistant Emile Duclaux, to the physiology course taught by Claude Bernard at the Sorbonne. There he took notes and humbly relived his years of training in the halls of the university. But he found it difficult to learn a whole new field; and indeed, since he had neither the time nor the patience to do this, he soon preferred to form his own ideas on the problem at hand.

Once again, Pasteur was successful in addressing a biological problem; this time bacteria infecting silkworms (they are not really a worm, but a caterpillar).

The caterpillar of Alés led Pasteur from microbiology to veterinary science to medicine… When Pasteur revolutionized the science of his era by discovering the germs and their role, it was only natural that he should become interested in medicine and hygiene.

At this point, Pasteur had essentially completed his transition from physics to biology and medicine. I won’t discuss his later work on the use of antiseptics in surgery, pasteurization, anthrax infection in sheep, or the development of a rabies vaccine. Debré summarizes,

In his last studies, Pasteur recalled that he had started out as a chemist. First in the laboratory of the rue d’Ulm and then in his Institute, his ultimate experiments indicate that he was trying to understand how the same microbe can either kill a person or stimulate his or her resistance. This is where bacteriology merged into immunology. Pasteur brought these neighboring disciplines together. Understanding the role of the molecules, the toxins, and the antitoxins involved both chemistry and biology.

So what do I conclude about Pasteur’s transition from the physical to the biological sciences? It wasn’t part of a long-range plan. Nor was it primarily motivated by the desire to help the sick, at least initially. I see two key points. First, the rotation of polarized light when passed through an organic substance led him naturally from physics to biology; scientific problems don’t always respect academic boundaries. Second, requests to address industrial problems further accelerated this transition, and those problems happened to be biological in nature. There seems to be a lot of chance involved in this transition (I think there often is for many scientists). But, as Pasteur famously said, chance favors the prepared mind. 

 

 

https://www.youtube.com/watch?v=OXdbQ1JkX7c
 

 https://www.youtube.com/watch?v=1lLNZQVPpQA

The Deadly Rise of Anti-Science

The Deadly Rise of Anti-Science,
by Peter Hotez.

This week I read The Deadly Rise of Anti-Science: A Scientist’s Warning, by Peter Hotez. Every American should read this book. In his introductory chapter, Hotez writes

This is a dark and tragic story of how a significant segment of the population of the United States suddenly, defiantly, and without precedent turned against biomedical science and scientists. I detail how anti-science became a dominant force in the United States, resulting in the deaths of thousands of Americans in 2021 and into 2022, and why this situation presents a national emergency. I explain why anti-science aggression will not end with the COVID-19 pandemic. I believe we must counteract it now, before something irreparable happens to set the country on a course of inexorable decline…

The consequences are shocking: as I will detail, more than 200,000 Americans needlessly lost their lives because they refused a COVID-19 vaccine and succumbed to the virus. Their lives could have been saved had they accepted the overwhelming scientific evidence for the effectiveness and safety of COVID-19 immunization or the warnings from the community of biomedical scientists and public health experts about the dangers of remaining unvaccinated. Ultimately, this such public defiance of science became a leading killer of middle-aged and older Americans, more than gun violence, terrorism, nuclear proliferation, cyberattacks or other major societal threats.

Where did this 200,000 number come from? On page 2 of Intermediate Physics for Medicine and Biology, Russ Hobbie and I claim that

One valuable skill in physics is the ability to make order-of-magnitude estimates, meaning to calculate something approximately right.

Hotez gives a classic example of estimation when deriving the 200,000 number. First, he notes that 245,000 Americans died of covid between May 1 and December 31, 2021. Covid arrived in the United States in early 2020, but vaccines did not become widely available until mid 2021. Actually, the vaccines were ready in early 2021 (I had my first dose on March 20), but May 1 was the date when the vaccine was available to everyone. During the second half of 2021, about 80% of Americans who died of covid were unvaccinated. So, Hotez multiplies 245,000 by 0.8 to get 196,000 unvaccinated deaths. After rounding this off to one significant figure, this is where he gets the number 200,000.

There are a few caveats. On the one hand, our estimate may be too high. The vaccine is not perfect. If all of the 200,000 unvaccinated people who died would have gotten the vaccine, some of them would still have perished from covid. If we take the vaccine as being 90% effective against death, we would multiple 196,000 times 0.9 to get 176,400. On the other hand, our estimate may be too low. Covid did not end on January 1, 2022. In fact, the omicron variant swept the country that winter and at its peak over 2000 people died of covid each day. So, the total covid deaths since the vaccine became available—the starting point of our calculation—is certainly higher than 245,000.

As Hotez points out, other researchers have also estimated the number of unnecessary covid deaths, using slightly different assumptions, and all the results are roughly consistent, around 200,000. (Hotez’s book appears to have been written in mid-to-late 2022; I suspect the long tail of covid deaths since then would not make much difference to this estimation, but I’m not sure.) 

In the spirit of an order-of-magnitude estimate, one should not place too great an emphasis on the precise number. It was certainly more than twenty thousand and it was without a doubt less than two million. I doubt we’ll ever know if the “true” amount is 187,000 or 224,000 or any other specific value. But we can say with confidence that about a couple hundred thousand Americans died unnecessarily because people were not vaccinated. Hotez concludes

That 200,000 unvaccinated Americans gave up their lives needlessly through shunning COVID-19 vaccines can and should haunt our nation for a long time to come.

Infectious disease scientists such as Peter Hotez, Tony Fauci, and others are true American heroes. That far-right politicians and journalists vilify these researchers is despicable and disgusting. We all owe these scientists so much. Last Monday was “Public Health Thank You Day” and yesterday was Thanksgiving. I can think of no one more deserving of our thanks than the scientists who led the effort to vaccinate America against covid. 

Why Science Isn’t Up for Debate, with Peter Hotez.

https://www.youtube.com/watch?v=PbGfeksduGE

Gustav Bucky and the Antiscatter Grid

An antiscatter grid.
Episcophagus, CC BY-SA 4.0,
via Wikimedia Commons.

In Chapter 16 of Intermediate Physics for Medicine and Biology, Russ Hobbie and I discuss the antiscatter grid used in radiography.

Since the radiograph assumes that photons either travel in a straight line from the point source in the x-ray tube to the detector or are absorbed, Compton-scattered photons that strike the detector reduce the contrast and contribute an overall background darkening. This effect can be reduced by placing an antiscatter grid (or radiographic grid, or “bucky” after its inventor, Gustav Bucky) just in front of the detector.

Who is Gustav Bucky? We can learn more about his life and work by examining the chapter “Two Centenaries: William Coolidge & Gustav Bucky,” by Elizabeth Beckmann and Adrian Thomas, in The Story of Radiology (Volume 2), published by the European Society of Radiology. Beckmann and Thomas begin

Gustav Peter Bucky was born on September 3, 1880 in Leipzig, Germany. He wanted to be an engineer, however at the insistence of his parents he transferred to study medicine at the University of Leipzig, graduating in1906. The combination of his interest in photography at school, his ambition to be an engineer and his parent’s insistence that he study medicine would lead him into the relatively new technical branch of medicine which was to be called radiology.

I’ve seen many reasons for scientists to straddle between physics/engineering and biology/medicine. In Bucky’s case the reason was parental pressure.

Beckmann and Thomas of course mention Bucky’s biggest contribution to science, his antiscatter grid.

It was Gustav Bucky who realised that the main problem was finding a way to reduce the scattered radiation that was responsible for the loss of definition of the radiological image from reaching the film. However, this had to be achieved with minimum impact on the primary x-ray beam. Bucky had his original idea on how to achieve this in 1909, but it took some years of experimenting for him to develop his design.

Bucky described his original design for the ‘Bucky Diaphragm’ as a ‘honeycomb’ lead grid, but with individual elements being square in shape, rather than hexagonal. He used lead since it was a material which absorbed x-rays. In this design the lead strips were thick and spaced 2 cm apart, running both parallel to the length and width of the film. This resulted in the lines of the grid being visible on the x-ray film. Despite this, the grid was effective and did remove scatter and improve image contrast.

You can eliminate those artifact lines by moving the grid.

In 1920, the American Hollis Potter further developed the grid. Potter aligned the lead strips so that they now ran in one direction only, and he also made the lead strips thinner so that they were less visible on the image. Potter also proposed moving the grid during exposure, which blurred out the image of the lead strips on the radiographic image... The resulting moving grid, based upon the work of Bucky and Potter, became known as the Potter-Bucky grid.

Albert Einstein and Gustav Bucky,
Leo Baeck Institute, F 5347B.

Bucky moved from Germany to the United States in 1929. He became good friends with Albert Einstein.

In 1933, Bucky met up again with his friend Albert Einstein when he arrived in New York. When on holiday together Gustav and Albert would go for a long walk together each day, discussing and developing new ideas…

Probably the most famous collaboration between Bucky and Einstein was the idea of ‘a light intensity self-adjusting camera’ with a US patent granted on October 27, 1936...

It is a sign of the close relationship between Bucky and Einstein that Bucky visited Einstein every day during his final illness and was at the hospital only hours before Einstein’s death in April 1955.

The story concludes

Gustav Bucky was a friendly, modest, undemanding person who made a lasting and significant contribution to radiology. For 21st century radiology the impact of the invention for which Gustav Bucky is most remembered – the Bucky Grid – continues. The grid is as important in modern digital detection systems, like computed radiography (CR) plates or digital radiography (DR) detector systems, as it was with x-ray film in the 1920s. 

Monet's Water Lilies

When my wife and I were in Paris several years ago we visited the Musée de l’Orangerie, where Claude Monet’s beautiful water lily murals are displayed. Monet (1840–1926) is the famous impressionist painter who, during the last decades of his life, painted lilies floating on the surface of the pond at his home in Giverny. I remember sitting in one of the oval rooms staring at these giant paintings. It was so quiet and peaceful.

Monet’s water lily murals in the Musée de l’Orangerie in Paris.
Brady Brenot, CC BY-SA 4.0 , via Wikimedia Commons.

Water lilies take advantage of some interesting physics. First, their stalks and leaves contain air pockets, reducing their average density and making them buoyant. Russ Hobbie and I compare the effect of buoyancy in terrestrial and aquatic animals. I quote this comparison below, but I have replaced the word “animals” by “plants”.

Plants are made up primarily of water, so their density is approximately 10³ kg m⁻³. The buoyant force depends on the plant’s environment. Terrestrial plants live in air, which has a density of 1.2 kg m⁻³. The buoyant force on terrestrial plants is very small compared to their weight. Aquatic plants live in water, and their density is almost the same as the surrounding fluid. The buoyant force almost cancels the weight, so the plant is essentially “weightless.” Gravity plays a major role in the life of terrestrial plants, but only a minor role for aquatic plants. Denny (1993) explores the differences between terrestrial and aquatic plants in more detail.

Another piece of physics important to water lilies is surface tension, a topic only briefly mentioned in the fifth edition of Intermediate Physics for Medicine and Biology, but which (spoiler alert!) may play a larger role in the sixth edition. The lily’s leaf is waxy, which repels water and enhances its ability to remain on the water-air surface. In addition, small cilia increase the surface area.

A last bit of physics has to do with the surface-to-volume ratio. Usually surface tension can’t support a large object, because its weight increases with the cube of its linear size, whereas the effect of surface tension increases with the object’s perimeter. Therefore, the impact of gravity increases with size more dramatically than does the impact of surface tension, so a large object sinks like a rock. The water lily’s leaf, however, is thin, and making the leaf larger increases its surface area but not its thickness. The weight only increases as the square of its linear size, not as the cube. If the leaf is large enough, gravity will still win out, but the leaf can be larger than you might expect and still float on the water surface.

Monet donated his water lily murals to France at the end of World War I, to create a place where people could reflect on those who gave their life for the nation. When visiting them, you can also contemplate the role of physics in medicine and biology.

Happy Veterans Day.

One of Monet’s water lily murals at the Musée de l’Orangerie.

Monet’s Water Lilies: Great Art Explained.

 https://www.youtube.com/watch?v=fd-Me3EBGYY&t=7s

The Golay Coil

Last week I introduced the Helmholtz coil and the Maxwell coil. The Maxwell coil is useful for creating the magnetic field gradient needed for magnetic resonance imaging. At the end of the post, I wrote

The Maxwell coil is great for producing the magnetic field gradient dB_z/dz needed for slice selection in MRI, but what coil is required to produce the gradients dB_z/dx and dB_z/dy needed during MRI readout and phase encoding? That, my friends, is a story for another post.

Today, I will finish the story.

First, let’s assume the gradient coils are all located on the surface of a cylinder. If this were a clinical MRI scanner, the person would lie on a bed that would be slid into the cylinder to get an image. The Maxwell coil consists of two circular coils, separated by a distance equal to the square root of three times the coil radius. The parts of the coil in the back that are hidden by the cylinder are shown as dashed. The two coils carry current in opposite directions, as shown below, creating a gradient dB_z/dz in the imaging region midway between the two coils on the axis of the cylinder.

To perform imaging, however, you need gradients in the x and y directions too. To create dB_z/dx, you typically use what is called a Golay coil. It consists of four coils wound on the cylinder surface as shown below. 

The mathematics to determine the details of this design is too complicated for this post. Suffice to so, it requires setting the third derivative of B_z with respect to x equal to zero. The resulting coils should each subtend an angle of 120°. Their inner loops should be separated by 0.778 cylinder radii, and their outer loops by 5.14 radii.

To create the gradient dB_z/dy, simply rotate the Golay coil by 90°, as shown below. 

So, to perform magnetic resonance imaging you need a nested set of three coils as shown below. 

The picture gets confusing with all the hidden lines. Here is how the set looks with the hidden parts of the coils truly hidden.

While this set of coils will produce linear magnetic field gradients in the central region, in state-of-the-art MRI scanners the coils are somewhat more complicated, with multiple loops corresponding to each loop shown above.

We all know who Helmholtz and Maxwell are, but who is Golay? Marcel J. E. Golay (1902-1989) was a Swiss scientist who came to the US to get his PhD at the University of Chicago and then stayed. He had a varied career, making fundamental advances in chromatography, information theory, and the detection of infrared light. He studied the process of shimming: making small adjustments to the magnetic field of a MRI scanner to make the static field more homogeneous. This work ultimately led to the design of gradient coils.

In Chapter 18 of Intermediate Physics for Medicine and Biology, Russ Hobbie and I discuss magnetic resonance imaging and the need for magnetic field gradients. In a nutshell, MRI converts magnetic field strength to spin precession frequency. By measuring this frequency, you can obtain information about magnetic field strength. A magnetic field gradient lets you map frequency to position, an idea which is at the heart of imaging using magnetic resonance.

The Helmholtz Coil and the Maxwell Coil

To do magnetic resonance imaging, you need a static magnetic field that is uniform and a switchable magnetic field that has a uniform gradient. How do you produce such fields? In this post, I explain one of the simplest ways: using a Helmholtz coil and a Maxwell coil.

Both of these are created using circular coils. The magnetic field B_z produced by a circular coil can be calculated using the law of Biot and Savart (see Chapter 8 of Intermediate Physics for Medicine and Biology)

where μ₀ is the permeability of free space (the basic constant of magnetostatics), I is the coil current, N is the number of turns, R is the coil radius, and z is the distance along the axis from the coil center.

The Helmholtz Coil

The Helmholtz coil consists of two circular coils in parallel planes, having the same axis and the same current in the same direction, that are separated by a distance d. Our goal will be to find the value of d that gives the most uniform magnetic field. By superposition, the magnetic field is 

 

To create a uniform magnetic field, we will perform a Taylor expansion of the magnetic field about the origin (z = 0). We will need derivatives of the magnetic field. The first derivative is

(The reader will have to fill in the missing steps when calculating these derivatives.) At z = 0, this derivative will go to zero. In fact, because the magnetic field is even about the z axis, all odd derivatives will be zero, regardless of the value of d.

The second derivative is

At z = 0, the two terms in the brackets are the same. Our goal is to have this term be zero, implying that the second order term in the Taylor series vanishes. This will happen if

or, in other words, d = R. This famous result says that for a Helmholtz pair the coil separation should equal the coil radius.

A Helmholtz coil produces a remarkably uniform field near the origin. However, it is not uniform enough for use in most magnetic resonance imaging machines, which typically have a more complex set of coils to create an even more homogeneous field. If you need a larger region that is homogeneous, you could always just use a larger Helmholtz coil, but then you would need more current to achieve the desired magnetic field at the center. A Helmholtz pair isn’t bad if you want to use only two reasonably sized coils.

The Maxwell Coil

The Helmholtz coil produces a uniform magnetic field, whereas the Maxwell coil produces a uniform magnetic field gradient. It consists of two circular coils, in parallel planes having the same axis, that are separated by a distance d, but which have current in the opposite directions. Again, our goal will be to find the value of d that gives the most uniform magnetic field gradient. The magnetic field is

The only difference between this case and that for the Helmholtz coil is the change in sign of the second term in the bracket. If z = 0, the magnetic field is zero. Moreover, the magnetic field is an odd function of z, so all even derivatives also vanish. The first derivative is

This expression gives us the magnitude of the gradient at the origin, but it doesn’t help us create a more uniform gradient. The second derivative is

This derivative is zero at the origin, regardless of the value of d. So, we have to look at the third derivative.

At z = 0, this will vanish if

or, in other words, d = √3 R = 1.73 R. Thus, the two coils have a greater separation for a Maxwell coil than for a Helmholtz coil. The Maxwell coil would be useful for producing the slice selection gradient during MRI (for more about the need for gradient fields in MRI, see Chapter 18 of IPMB).

Conclusion

Below is a plot of the normalized magnetic field as a function of z for the Helmholtz coil (blue) and the Maxwell coil (yellow). As you can see, the region with a uniform field or gradient is small. It depends on what level of accuracy you need, but if you are more than half a radius from the origin you will see significant deviations from homogeneity.
 

Russ Hobbie and I never discuss the Helmholtz coil in Intermediate Physics for Medicine and Biology. We don’t mention the Maxwell coil by name, but Problem 33 of Chapter 18 analyzed a Maxwell pair even if we don’t call it that.

The Maxwell coil is great for producing the magnetic field gradient dB_z/dz needed for slice selection in MRI, but how do you produce the gradients dB_z/dx and dB_z/dy needed during MRI readout and phase encoding? That, my friends, is a story for another post.

Mr. Clough

A teacher affects eternity; he can never tell where his influence stops. 

Henry Adams

Stephen Clough, from the 1975
Homestead Jr.-Sr. High School Yearbook.

How does someone end up being coauthor on a textbook like Intermediate Physics for Medicine and Biology? It takes a lot of friends, teachers, and role models who help you along the way. I had many excellent teachers when I was young. One of the best was Stephen Clough.

I attended grades 7–10 at Homestead Junior-Senior High School. Usually a junior high and senior high are in separate buildings, but the suburb of Fort Wayne where I lived at the time was new and growing, and had the two combined. For two years (I think grades 9 and 10) I had English with Mr. Clough. He was one of the younger teachers and had longish hair and a mustache, and I thought he was little bit of a hippie. That’s OK, because in the mid 70s hippies were still groovy (although they would go out of fashion soon).

Before I had Mr. Clough, I didn’t read much. I was obsessed with baseball and would read an occasional sports biography, but not much else. I did well in school, but I don’t remember our classes being too challenging or having much homework. Life was about hanging around with friends, playing ping pong, riding bikes, listening to music, and watching television. But Mr. Clough had us reading modern fiction, like Animal Farm and Lord of the Flies. For me, this was an intellectual awakening. Before Mr. Clough I rarely read books; after Mr. Clough I read all the time (and still do).

Me (age 15) from the 1975
Homestead Jr.-Sr. High School Yearbook.

I remember how, on Fridays, Mr. Clough would bring his guitar to school and play for us and sing. I thought this was the coolest thing I’d ever seen. None of my other teachers related to us like that. He played a lot of Dylan. I’ll never forget the day he explained what the words meant in the song American Pie. 

Mr. Clough had a huge influence on my academic development. Reading books led to reading the scientific writing of Isaac Asimov, which led to majoring in physics in college, which led to a PhD, which ultimately led to becoming a coauthor of Intermediate Physics for Medicine and Biology. I owe him much.

As Henry Adams said, a teacher affects eternity. I hope everyone teaching a class using IPMB keeps that in mind. You can never tell where your influence stops. 

I last saw Mr. Clough at my 30^th high school reunion. My friend from high school, Dave Small, became an opera singer, and he sang several songs for us at the gathering. Guess who accompanied him on the guitar? Stephen Clough.

American Pie, by Don McLean.

https://www.youtube.com/watch?v=PRpiBpDy7MQ

J. Robert Oppenheimer, Biological Physicist

J. Robert Oppenheimer.

Did you watch Oppenheimer in the theater this summer? I did. The movie told how J. Robert Oppenheimer led the Manhattan Project that built the first atomic bomb during World War II. But the movie skipped Oppenheimer’s research in biological physics related to photosynthesis.

Russ Hobbie and I only make a passing mention of photosynthesis in Chapter 3 of Intermediate Physics for Medicine and Biology.

The creation of glucose or other sugars is the reverse of the respiration process and is called photosynthesis. The free energy required to run the reaction the other direction is supplied by light energy.

From Photon to Neuron,
by Philip Nelson.

To learn more about Oppie and photosynthesis, I turn to Philip Nelson’s wonderful textbook From Photon to Neuron: Light, Imaging, Vision. His discussion of photosynthesis begins

Photosynthetic organisms convert around 10¹⁴ kg of carbon from carbon dioxide into biomass each year. In addition to generating the food that we enjoy eating, photosynthetic organisms emit a waste product, free oxygen, that we enjoy breathing. They also stabilize Earth’s climate by removing atmospheric CO₂.

Nelson begins the story by introducing William Arnold, Oppenheimer’s future collaborator.

W. Arnold was an undergraduate student interested in a career in astronomy. In 1930, he was finding it difficult to schedule all the required courses he needed for graduation. His advisor proposed that, in place of Elementary Biology, he could substitute a course on Plant Physiology organized by [Robert] Emerson. Arnold enjoyed the class, though he still preferred astronomy. But unable to find a place to continue his studies in that field after graduation, he accepted an offer from Emerson to stay on as his assistant.

Emerson and Arnold went on to perform critical experiments on photosynthesis. Then Emerson performed another experiment with [Charlton] Lewis, in which they found that chlorophyll does not absorb light with a wavelength of 480 nm (blue), but an accessory pigment called phycocyanin does. Emerson and Lewis concluded that “the energy absorbed by phycocyanin must be available for photosynthesis.”

Here is where Oppenheimer comes into the story. I will let Nelson tell it.

Could phycocyanin absorb light energy and somehow transfer it to the chlorophyll system?...

Arnold eventually left Emerson’s lab to study elsewhere, but they stayed in contact. Emerson told him about the results with Lewis, and suggested that he think about the energy-transfer problem. Arnold had once audited a course on quantum physics, so he visited the professor for that course to pose the puzzle. The professor was J. R. Oppenheimer, and he did have an idea. Oppenheimer realized that a similar energy transfer process was known in nuclear physics; from this he created a complete theory of fluorescence resonance energy transfer. Oppenheimer and Arnold also made quantitative estimates indicating that phycocyanin and chlorophyll could play the roles of donor and acceptor, and that this mechanism could give the high transfer efficiency needed to explain the data.

So, what nuclear energy transfer process was Oppenheimer talking about? In Arnold and Oppenheimer’s paper, they wrote

It is the purpose of the present paper to point out a mechanism of energy transfer from phycocyanin to chlorophyll, the efficiency of which seems to be high enough to account for the results of Emerson and Lewis. This new process is, except for the scale, identical with the process of internal conversion that we have in the study of radioactivity.

Internal conversion is a topic Russ and I address in IPMB. We said

Whenever a nucleus loses energy by γ decay, there is a competing process called internal conversion. The energy to be lost in the transition, E_γ, is transferred directly to a bound electron, which is then ejected.

Introductory Nuclear Physics,
by Kenneth Krane.

More detail can be found in Introductory Nuclear Physics by Kenneth Krane.

Internal conversion is an electromagnetic process that competes with γ emission. In this case the electromagnetic multipole fields of the nucleus do not result in the emission of a photon; instead, the fields interact with the atomic electrons and cause one of the electrons to be emitted from the atom. In contrast to β decay, the electron is not created in the decay process but rather is a previously existing electron in an atomic orbit. For this reason internal conversion decay rates can be altered slightly by changing the chemical environment of the atom, thus changing somewhat the atomic orbits. Keep in mind, however, that this is not a two-step process in which a photon is first emitted by the nucleus and then knocks loose an orbiting electron by a process analogous to the photoelectric effect; such a process would have a negligibly small probability to occur.

Nelson compares the photosynthesis process to another process widely used in biological imaging: Fluorescence resonance energy transfer (FRET). He describes FRET this way.

We can find pairs of molecular species, called donor/acceptor pairs, with the property that physical proximity abolishes fluorescence from the donor. When such a pair are close, the acceptor nearly always pulls the excitation energy off the donor, before the donor has a chance to fluoresce. The acceptor may either emit a photon, or lose its excitation without fluorescence (“nonradiative” energy loss).

Let’s put this all together. The donor in FRET is like the phycocyanin molecule in photosynthesis is like the nucleus in internal conversion. The acceptor in FRET is like the chlorophyll molecule in photosynthesis is like the electron cloud in internal conversion. The fluorescence of the donor/phycocyanin/nucleus is suppressed (in the nuclear case, fluorescence would be gamma decay). Instead, the electromagnetic field of the donor/phycocyanin/nucleus interacts with, and transfers energy to, the acceptor/chlorophyll/electron cloud. In the case of FRET, the acceptor then fluoresces (which is what is detected when doing FRET imaging). The chlorophyll/electron cloud does not fluoresce, but instead ejects an electron in the case of internal conversion, or energizes an electron that can ultimately perform chemical reactions in the case of photosynthesis. All three processes are exquisitely sensitive to physical proximity. For FRET imaging, this sensitivity allows one to say if two molecules are close to each other. In photosynthesis, it means the chlorophyll and phycocyanin must be near one another. In internal conversion, it means the electrode cloud must overlap the nucleus, which implies that the process usually results in emission of a K-shell electron since those innermost electrons have the highest probability of being near the nucleus.

There’s lots of interesting stuff here: How working at the border between disciplines can result in breakthroughs; how physics concepts can contribute to biology; how addressing oddball questions arising from data can lead to new breakthroughs; how quantum mechanics can influence biological processes (Newton rules biology, except when he doesn’t); how seemingly different phenomena—such as FRET imaging, photosynthesis, and nuclear internal conversion—can have underlying similarities. I wish my command of quantum mechanics was strong enough that I could explain all these resonance effects to you in more detail, but alas it is not.

Oppenheimer and General Groves
at the Trinity test site. I love
Oppie’s pork pie hat.

If you haven’t seen Oppenheimer yet, I recommend you do. Go see Barbie too. Make it a full Barbenheimer. But if you want to learn about the father of the atomic bomb’s contributions to biology, you’d better stick with From Photon to Neuron or this blog. 

 

 

The official trailer to Oppenheimer.

https://www.youtube.com/watch?v=bK6ldnjE3Y0

 

 

Photosynthesis.

https://www.youtube.com/watch?v=jlO8NiPbgrk&t=14s

The Dobson Unit

In Chapter 14 of Intermediate Physics for Medicine and Biology, Russ Hobbie and I discuss the risk of DNA damage—and therefore cancer—caused by ultraviolet light from the sun. Figure 14.28 in IPMB presents the results of a calculation of UV dose rate, weighted for DNA damage. The caption of the figure states “the calculation assumes clear skies and an ozone layer of 300 Dobson units (1 DU = 2.69 × 10²⁰ molecule m^-2).”

The Dobson Unit, what’s that?

Rather than explaining it myself, let me quote the NASA website about ozone.

What is a Dobson Unit?

The Dobson Unit is the most common unit for measuring ozone concentration. One Dobson Unit is the number of molecules of ozone [O₃] that would be required to create a layer of pure ozone 0.01 millimeters thick at a temperature of 0 degrees Celsius and a pressure of 1 atmosphere (the air pressure at the surface of the Earth). Expressed another way, a column of air with an ozone concentration of 1 Dobson Unit would contain about 2.69 × 10¹⁶ ozone molecules for every square centimeter of area at the base of the column. Over the Earth’s surface, the ozone layer’s average thickness is about 300 Dobson Units or a layer that is 3 millimeters thick.

The Dobson Unit was named after British physicist and meteorologist Gordon Miller Bourne Dobson (1889 –1976) who did early research on ozone in the atmosphere.

Worried about climate change? The ozone story may provide some hope. When man-made chemicals such as chlorofluorocarbons, for example freon, are released into the atmosphere, they damage the ozone layer, allowing larger amounts of ultraviolet radiation to reach the earth’s surface. In the 1970s, an ozone hole developed each year over the south pole. In 1987, countries from all over the world united to pass the Montreal Protocol, which banned many ozone depleting substances. Since that time, the ozone hole has been getting smaller. This represents a success story demonstrating how international cooperation can address critical environmental hazards. Now, we need to do the same thing for greenhouse gases to combat climate change. 

 

How the ozone layer was discovered.

https://www.youtube.com/watch?v=GS0dilngPws

Don't let this happen to your planet!

https://www.youtube.com/watch?v=nCpH71npnvo