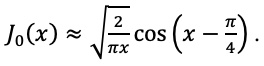The Greatest Shortcoming of the Human Race is our Inability to Understand the Exponential Function

Ten years ago yesterday, on September 7, 2013, Physicist Albert Bartlett died at the age of 90. Russ Hobbie and I mentioned Bartlett in Chapter 2 of Intermediate Physics for Medicine and Biology.

Exponential growth cannot go on forever. This fact is often ignored by economists and politicians. Albert Bartlett has written extensively on this subject. You can find several references in The American Journal of Physics and The Physics Teacher. See the summary in Bartlett (2004).

The Essential Exponential!
by Albert Bartlett.

The reference is to

Bartlett A (2004) The Essential Exponential! For the Future of our Planet. Center for Science, Mathematics & Computer Education, Lincoln

To celebrate Bartlett’s life, I’ll quote excerpts from an interview with Paul Nachman in May, 2005.

I started as an undergraduate in the fall of 1940 at Otterbein College in Westerville, Ohio. (It's a small, church-founded school, and my father was a Professor of Education there. Westerville back then was a separate town north of Columbus. Now it's been engulfed by Columbus.) In the spring of 1941, I was looking for some adventure, so I got a summer job washing dishes on an iron ore freighter on the Great Lakes. It took me several hitchhiking trips to Cleveland to get all the federal papers you need to work on the ships…

When September came along, I was having a good time, making good money, so I didn’t return to school. And that fall was Pearl Harbor. We were unloading the last cargo of iron ore for the season at the Wickwire-Spencer steel mill in Tonawanda, New York, on December 7. I’d finished cleaning up after lunch, then gone to my room and turned on the radio. I was the first person on board to hear the report...

Fortunately for all of us, Bartlett returned to college.

I said to myself, “I need to get back to college.” Writing from the boat, I applied to transfer to Colgate University in upstate New York. I was accepted, so, coming into Cleveland one day, I told the steward I was leaving to go back to college. I hitchhiked back to my home, changed from shipboard clothes to college clothes, hitchhiked up to Hamilton, New York, and started there in the fall semester of 1942.

Bartlett studied physics and math, and graduated from Colgate in 1944. Listen to how he ended up working on the Manhattan Project.

One of my professors heard that there was an address, “Box 1663,” in Santa Fe, New Mexico, where they were hiring physicists. Sounded like adventure to me, so I applied. Didn’t know what it was, and they didn't tell me and I was accepted. 

My only instructions were to appear at 109 E. Palace in Santa Fe. So, after graduation, I hitchhiked home to Ohio, and to Springfield where a friend helped me get two new International Harvester trucks from the factory. I drove them to a dealer in Oklahoma City and then hitchhiked to Amarillo. All my life I’d wanted to ride a freight train, so I hopped a freight in Amarillo to Belen, south of Albuquerque. Then I hitchhiked to Santa Fe and reported to 109 E. Palace. The lady there in the office was Dorothy McKibben. She did some paperwork and had me get on an Army bus in the alley in back. It took me off through the desert and canyons up to Los Alamos. Quite an experience! I arrived on July 18, 1944 and worked for 25 months there.”

At Los Alamos he worked on mass spectrometry of plutonium.

It wasn’t that I knew anything about mass spectrometry, but this was just at a time when the first plutonium was coming down from Hanford, and there was some indication that, in addition to the principal isotope ²³⁹Pu, there may be some ²⁴⁰Pu from an extra neutron capture in the Hanford reactors.

Now ²⁴⁰Pu has a high spontaneous fission probability, which would upset all their [bomb] calculations. So they wanted to know how much was there. They’d just requisitioned a mass spectrometer from a lab in Washington, DC, about the time I got there, and Bob Thompson, who’d been a PhD student with Al Nier at Minnesota, was setting it up. Bob took me on me sort of as an apprentice and took a real interest in me, telling me what I needed to study, giving me a stack of reprints to read, etc. He was very helpful. So we made the first measurements of the isotopic constitution of plutonium coming out of the reactors.

In 1946 he went to Harvard for graduate study in physics. His PhD project was to build

… a beta-ray [electron] spectrometer. I finished at Harvard and came out to Colorado in the late summer of 1950. I’ve been here ever since.

By Colorado, he meant the University of Colorado in Boulder. I interviewed at Boulder for graduate school around 1982. I can’t remember if I met Bartlett. I hope I did.

Bartlett was primarily focused on physics education. He was a leader in the American Association of Physics Teachers.

In 1978, I was national President of the American Association of Physics Teachers [AAPT]. I had four years in the AAPT presidential sequence vice-president, president-elect, president, and past president with duties in each of those offices. In ’78 I was the President, so I was quite involved with physics education, and I think that was why I was named an APS Fellow.

Bartlett is best known for his talk on exponential growth and the environment, which he gave over 1700 times (watch in on the YouTube videos below). Here is how it all got started.

Well, during all the uprising on the campus, there was a surge of student interest in the environment, and I had been slowly coming to the realization that students and other people didn’t have an understanding of the arithmetic of compound interest. So I started putting some notes together, and in September of 1969 the undergraduate pre-med honor society asked if I could speak on something or other at one of their meetings. I’d known many of these kids from the previous year when I was lecturing in the pre-med beginning physics course, so I put these notes together and on September 19, 1969 talked to them about growth and the problems of growth.

It didn’t have anything like the scope of today’s presentation. But, fortunately, I kept my notes and the next year I had two or three more invitations. It evolved as I became more interested. Finally in '78 or '79, I gave the talk 131 times. It was sort of like a Hubbert peak! It went down after that, but instead of going to zero, it’s leveled off at about 40 times a year.

With climate change becoming the major environmental issue of our time, we must all remember Bartlett’s famous quote:

The greatest shortcoming of the human race is our inability to understand the exponential function.

This inability is all the more reason to study Chapter 2 in IPMB, about exponential growth and decay. The survival of our species may depend on us somehow learning to understand exponential growth and its limitations.

A final reason to celebrate Bartlett is that this year, 2023, is the 100th anniversary of his birth: March 21, 1923. Happy 100th birthday, Albert Bartlett. We need you now more than ever!

The Most Important Video You Will Ever See (Part 1)

The Most Important Video You Will Ever See (Part 2)

 

 The Most Important Video You Will Ever See (Part 3)

 

The Most Important Video You Will Ever See (Part 4)

 The Most Important Video You Will Ever See (Part 5)

 

 

The Most Important Video You Will Ever See (Part 6)

 

 

The Most Important Video You Will Ever See (Part 7)

 

 

The Most Important Video You Will Ever See (Part 8)

 

Al Bartlett discusses population growth, climate change, energy, and consumption.

Is Japan's Plan to Release Radioactive Water into the Pacific Ocean Safe?

When listening to NBC Nightly News on August 24, I heard Lester Holt discuss Japan’s plan to release into the Pacific Ocean treated wastewater from the Fukushima nuclear power plant, the reactor that melted down after the 2011 earthquake and tsunami. Apparently this plan has caused an uproar, with Russia banning the import of seafood from Japan and residents of South Korea staging protests.

Is there a significant risk to dumping this treated water into the ocean? I will base my analysis on the recently published IAEA Comprehensive Report on the Safety Review of the ALPS-Treated Water at the Fukushima Daiichi Nuclear Power Station.

Two years ago, Japan developed a plan for the handling of Advanced Liquid Processing System (ALPS) treated water at the Fukushima Nuclear Power Station, which included a proposal to release the water into the ocean. Japan then asked the International Atomic Energy Agency (IAEA) to review the safety of their plan. The IAEA Director General established a task force to conduct this review, consisting of independent experts from all over the world (including Russia and South Korea). The task force recently published its report, whose purpose is “to present the IAEA’s final conclusions and findings of the technical review to assess whether the planned operation to discharge the ALPS treated water into the Pacific Ocean over the coming decades is consistent with relevant international safety standards.” The task force concluded that “the approach to the discharge of ALPS treated water into the sea… [is] consistent with relevant international safety standards” and that “the discharge of the ALPS treated water… will have a negligible radiological impact on people and the environment.”

In Chapter 16 of Intermediate Physics for Medicine and Biology, Russ Hobbie and I analyze the risk of radiation. We write

One way to express risk is to compare medical doses to the natural background. We are continuously exposed to radiation from natural sources. These include cosmic radiation, which varies with altitude and latitude; rock, sand, brick, and concrete containing varying amounts of radioactive minerals; the naturally occurring radionuclides in our bodies such as ¹⁴C and ⁴⁰K; and radioactive progeny from radon gas.

The effective dose of radiation is measured in sieverts, or more conveniently millisieverts (mSv). The typical effective dose from natural sources is about 3 mSv per year. What is the dose expected from releasing Japan’s radioactive water into the Pacific? According to the IAEA report, it is in the range from 0.000002 to 0.00004 mSv per year. So, we constantly are exposed to about 3 mSv/year of radiation and now we will experience 3.00004 mSv/year. The risk is negligible.

According to Table 16.6 in IPMB, flying in a plane at 40,000 feet—where cosmic ray exposure is increased—is 0.007 mSv per hour. That means the extra dose caused by the release of radioactive water is approximately equal to the extra dose received during 20 seconds of airplane flight. 

The value of 0.00004 mSv/year assumes the water is slowly released as planned. What if there is an accident? The IAEA report examined two accident scenarios and concluded that the upper limit of exposure is 0.01 mSv per accident. In other words, if three of the holding tanks accidentally dump all of their treated water into the Pacific at one time, your dose would be less than one percent of your yearly dose from natural sources. 

Another way to look at it the risk is to analyze the amount of tritium released. The treatment of the water before release removes most of the radioactive contaminates except tritium, which is the radioactive isotope hydrogen-3. Tritium is usually found as part of a water molecule, so it is extraordinarily difficult to separate it form normal water. Tritium is constantly being created in our atmosphere by cosmic rays colliding with nitrogen. About 100,000 TBq is produced each year. A becquerel (Bq) is one nuclear decay and tera- (T) is the metric prefix for 1,000,000,000,000. How much tritium will be released each year from Japan’s wastewater? 22 TBq. In other words, the amount of tritium released is about 5000 times less than the amount naturally produced. Once the released water is diluted and mixed with ocean water, the increase in tritium concentration will be insignificant.

My conclusion is that releasing the treated water into the ocean is safe, with a large margin of safety. Russia and South Korea can relax. Lester Holt, I love ya, but you really gotta read the IAEA report more carefully. 

There are plenty of things we all should worry about (just listen to the rest of the news report in the video below). The release of Japan’s wastewater into the ocean is not one of them. 

NBC Nightly News with Lester Holt, August 24, 2023. See 14:30–16:40 for the report on Japan’s plan to release radioactive water into the ocean.

 https://www.youtube.com/watch?v=pdFrCE3VzVQ

Oppose Federal Legislation That Would Protect Homeopathic Drugs From FDA Regulation

In Intermediate Physics for Medicine and Biology, Russ Hobbie and I don’t talk about homeopathy (thank goodness!). A homeopathic medicine is one that has been diluted with water multiple times (for example, 30 dilutions, each by a factor of ten), until not even a single molecule of the active ingredient remains. Proponents of homeopathy believe that the water “remembers” the original ingredient. This, of course, conflicts with everything scientists know about water. If you believe physics underlies medicine, you should reject homeopathy.

Why bring up homeopathy now? I recently received an email from one of my favorite organizations—the Center For Inquiry (CFI)—calling on people to oppose federal legislation that would limit the Food and Drug Administration’s ability to regulate homeopathic drugs. Rather than repeating everything the CFI said, I’ll simply quote from their website. I already wrote my Congressman about this issue.

CFI calls on our supporters to help defeat a pro-homeopathy amendment being proposed for the federal appropriations bill H.R. 4368. The homeopathy lobby is pushing hard for this amendment, and we need CFI supporters to voice their opposition to their members of Congress.

Homeopathy groups such as Americans For Homeopathy Choice (AFHC) are lobbying strenuously for Appropriations Amendment #4. This amendment would bar FDA enforcement of the Food, Drug, and Cosmetic Act against new homeopathic drug products as long as a product complies with “standards for strength, quality, and purity set forth in the Homeopathic Pharmacopoeia of the United States.” In other words, it would replace much-needed federal regulation with the industry’s own standards.

CFI has consistently pointed out that homeopathy is bunk science that does not work beyond the placebo effect. Homeopathic products are typically diluted to the point that no active ingredients remain. It is quack medicine and consumer fraud.

The Homeopathic Pharmacopoeia’s standards of quality are not medically valid. Yet the amendment would exempt homeopathic products from FDA regulation and oversight if they comport with those standards. This amounts to an argument of “No need for federal regulation, we can regulate ourselves with our own standards even if they constitute medical fraud” – or, more succinctly, “Let the fox guard the henhouse, please.” (Indeed, CFI has tussled with the Homeopathic Pharmacopoeia before.)

At the moment, AFHC and the homeopathy lobby are seeking additional co-sponsors in the House of Representatives for their amendment. This is where CFI’s supporters come in.

We need our supporters to mobilize and contact their members in the House of Representatives immediately. Please let them know, in no uncertain terms, that homeopathy cannot and must not escape federal regulation. It is crucial to keep Appropriations Amendment #4 out of the federal appropriations bill.

 

 Homeopathy, quackery and fraud, a TED talk by James Randi.

https://www.youtube.com/watch?v=c0Z7KeNCi7g

Paul Maccabee (1944–2023)

Paul Maccabee (1944–2023).
Photo used with permission from the
Downstate Health Sciences University website.

My friend and collaborator Paul Maccabee died on July 24. Paul was a pioneer in the field of magnetic stimulation, a topic that Russ Hobbie and I discuss in Chapter 8 of Intermediate Physics for Medicine and Biology. Paul’s career and mine had many parallels. We both worked on magnetic stimulation in the late 1980s and early 1990s. We both collaborated with a leading neurophysiologist: Paul with Vahe Ammasian and me with Mark Hallett. We both recognized the importance of laboratory animal experiments for identifying physiological mechanisms. We both were comfortable working with biomedical engineers, I entered that field from physics and Paul from medicine. 

Paul was about 15 years older than me and I viewed him as a role model. I believe I first met him at the 1989 International Motor Evoked Potential Symposium in Chicago, a key early conference dedicated to magnetic stimulation. Our paths crossed at other scientific meetings and his research had a major impact on my own. For years I taught a graduate class on bioelectricity at Oakland University and I had my students read Paul’s 1993 Journal of Physiology paper (described below) which I assigned because it’s a classic example of a well-written scientific article. According to Google Scholar that paper has been cited 374 times, and it should be cited even more.

I wrote about Paul in my review of the development of transcranial magnetic stimulation (BOHR International Journal of Neurology and Neuroscience, Volume 1, Pages 8–20, 2022, https://doi.org/10.54646/bijnn.002). Below I quote part of that article. I put his name in bold so you can find it easily.

Although this experiment [performed by Jan Nilsson and Marcela Panizza at the National Institutes of Health, see reference 49] confirmed [Peter Basser and my] prediction [that neural excitation occurs where the gradient of the induced electric field is largest, see reference 58], there were nevertheless concerns because of the heterogeneous nature of the bones and muscles in the human arm. At about the same time Nilsson and Panizza were doing their experiment at NIH, Paul Maccabee was performing an even better experiment at the New York Downstate Medical Center in Brooklyn. Maccabee obtained his MD from Boston University and collaborated in Brooklyn with the internationally acclaimed neuroscientist Vahe Ammasian [1, 40–43]. This research culminated in their 1993 article in the Journal of Physiology, in which they examined magnetic stimulation of a peripheral nerve lying in a saline bath [44]. First, they measured the electric field E_y (they assumed the nerve would lie above the coil along the y-axis) and its derivative along the nerve produced by a figure-8 coil located under the bath (Figure 9). They found that the electric field was maximum directly under the center of the coil, but the magnitude of the gradient dE_y/dy was maximum a couple centimeters either side of the center.

Figure 9. Contour plots of the electric field (E_y, red) and its spatial derivative (dE_y/dy, blue) induced by a figure-eight coil (purple) placed under a tank filled with saline and measured using a bipolar recording electrode. The y direction is downward in the figure, parallel to the direction of the nerve (see Figure 10). Adapted from Figure 2 of Maccabee et al. [44].

Next they placed a bullfrog sciatic nerve in the dish and recorded the electrical response from one end (Figure 10). They found a 0.9 ms delay between the recorded action potentials when the polarity of a magnetic stimulus was reversed (the yellow and red traces on the right). Given a propagation speed of about 40 m/s, the shift in excitation position was about 3.6 cm, consistent with what Basser and I would predict.

Figure 10. Recordings from an electrode (black dot) at the distal end of a bullfrog sciatic nerve (green) that was immersed in a trough filled with saline (blue) and stimulated with a figure-8 coil (purple). The nerve emerged from the saline to rest on the recording electrode in air. The compound nerve action potentials were elicited by a stimulus of one polarity (orange), then the other (red). Adapted from Figure 3 of Maccabee et al. [44].

So far, their study was similar to what we performed at NIH in a human, but then they did an experiment that we could not do. To determine how a heterogeneity would impact their results, they placed two insulating cylinders on either side of the nerve (Figure 11). These cylinders modified the electric field, moving the negative and positive peaks of the activating function closer together. They observed a corresponding reduction in the latency shift. This experiment provided insight into what happens when a human nerve passes between two bones, or some similar heterogeneity.

Figure 11. Magnetic stimulation of a sheep phrenic nerve immersed in a homogeneous (left) and inhomogeneous (right) volume conductor. The figure-8 coil (purple) was positioned under the nerve (green). The yellow circles indicate the position of the insulating cylinders. The electric field E_x (red) and its gradient dE_x/dx (blue) were measured along the nerve trajectory. The compound nerve action potentials at the recording electrode were measured for a magnetic stimulus of one polarity (orange) and then the other (green). Adapted from Figure 5 of Maccabee et al. [44].

Finally, they changed the experiment by bending the nerve and found that a bend caused a low threshold “hot spot,” and that excitation at that spot occurred where the electric field, not its gradient, was large. This result was consistent with Nagarajan and Durand’s analysis of excitation of truncated nerves [47].

In my opinion, Maccabee’s [44] article is the most impressive publication in the magnetic stimulation literature. Only Barker’s original demonstration of transcranial magnetic stimulation can compete with it [2].

Later in that review, I discussed a collaborative paper that Paul and I published.

One frustrating feature of the activating function approach is that excitation does not occur directly under the center of a figure-8 coil, where the electric field is largest, but off to one side, where the gradient peaks (Figure 9). Medical doctors do not want to guess how far from the coil center excitation occurs; they would prefer a coil for which “x” marks the spot. It occurred to me that such a coil could be designed using two adjacent figure-8 coils. I called this the four-leaf coil (Figure 12). John Cadwell from Cadwell Laboratories (Kennewick, Washington) built such a coil for me. Having seen the excellent results that Maccabee was obtaining using his nerve-in-a-dish setup, I sent the coil to him so he could test it. The resulting paper [65] showed that for one polarity of the stimulus the magnitude of the gradient of the electric field was largest directly under the coil center so the axons there were depolarized (“x” really did mark the spot of excitation). In addition, if the polarity of the stimulus was reversed, the magnitude of the gradient remained large under the coil center, but it now tended to hyperpolarize rather than depolarize the axons. Maccabee and I hoped that such hyperpolarization could be used to block action potential propagation, acting like an anesthetic. The Brooklyn experiments verified all the predictions of the activating function model for the four-leaf coil. Unfortunately, Maccabee never observed any action potential block. Perhaps, the hyperpolarization required for block was greater than the coil could produce.

Figure 12. A four-leaf coil (purple) used to stimulate a peripheral nerve (blue). Adapted from Figure 1 of Roth et al. [65].

[1] Amassian, V. E., Eberle, L., Maccabee, P. J., and Cracco, R. Q. (1992). Modelling magnetic coil excitation of human cerebral cortex witha peripheral nerve immersed in a brain-shaped volume conductor:The significance of fiber bending in excitation. Electroenceph. Clin. Neurophysiol., 85, 291–301.

[2] Barker, A. T., Jalinous, R., and Freeston, I. L. (1985). Non-invasive magnetic stimulation of human motor cortex. Lancet, 1, 1106–1107.

[40] Maccabee, P. J., Amassian, V. E., Cracco, R. Q., and Cadwell, J. A. (1988). An analysis of peripheral motor nerve stimulation in humans using the magnetic coil. Electroenceph. Clin. Neurophysiol., 70, 524–533.

[41] Maccabee, P. J., Eberle, L., Amassian, V. E., Cracco, R. Q., Rudell, A., and Jayachandra, M. (1990). Spatial distribution of the electric field induced in volume by round and figure ‘8’ magnetic coils: Relevance to activation of sensory nerve fibers. Electroenceph. Clin. Neurophysiol., 76, 131–141.

[42] Maccabee, P. J., Amassian, V. E., Cracco, R. Q., Cracco, J. B., Eberle, L., and Rudell, A. (1991a). Stimulation of the human nervous system using the magnetic coil. J. Clin. Neurophysiol., 8, 38–55.

[43] Maccabee, P. J., Amassian, V. E., Eberle, L. P., Rudell, A. P., Cracco, R. Q., Lai, K. S., and Somasundarum, M. (1991b). Measurement of the electric field induced into inhomogeneous volume conductors by magnetic coils: Application to human spinal neurogeometry. Electroenceph. Clin. Neurophysiol., 81, 224–237.

[44] Maccabee, P. J., Amassian, V. E., Eberle, L. P., and Cracco, R. Q. (1993). Magnetic coil stimulation of straight and bent amphibian and mammalian peripheral nerve in vitro: Locus of excitation. J. Physiol., 460, 201–219.

[47] Nagarajan, S. S., Durand, D. M., and Warman, E. N. (1993). Effects of induced electric fields on finite neuronal structures: A simulation study. IEEE Trans. Biomed. Eng., 40, 1175–1188.

[49] Nilsson, J., Panizza, M., Roth, B. J., Basser, P. J., Cohen, L. G., Caruso, G., and Hallett, M. (1992). Determining the site of stimulation during magnetic stimulation of a peripheral nerve. Electroenceph. Clin. Neurophysiol., 85, 253–264.

[58] Roth, B. J. and Basser, P. J. (1990). A model of the stimulation of a nerve fiber by electromagnetic induction. IEEE Trans. Biomed. Eng., 37, 588–597.

[65] Roth, B. J., Maccabee, P. J., Eberle, L., Amassian, V. E., Hallett, M., Cadwell, J., Anselmi, G. D., and Tatarian, G. T. (1994a). In-vitro evaluation of a four-leaf coil design for magnetic stimulation of peripheral nerve. Electroenceph. Clin. Neurophysiol., 93, 68–74.

Although my name was listed first on our joint 1994 article, Paul could easily have been the lead author. The coil shape was my idea but he performed all the experiments. I never set foot in Brooklyn; I just mailed the coil to him.

Paul was a giant in the field of magnetic stimulation. The articles I list above are only a few of the many he published. For a medical doctor he had a strong grasp of electricity and magnetism. I lost track of him over the years but had the good fortune to reconnect with him a few months ago by email.

I miss Paul Maccabee. Anyone who studies, uses, or benefits from magnetic stimulation owes him a debt of gratitude. I know I do.

The Connect Our Parks Act is Safe, but Maybe Not Wise

Congress is currently considering the “Connect Our Parks Act.” It is

a bill to require the Secretary of the Interior to conduct an assessment to identify locations in National Parks in which there is the greatest need for broadband internet access service and areas in National Parks in which there is the greatest need for cellular service, and for other purposes.

I don’t want people hiking through Yellowstone while squawking on their cell phone, so I’m not sure I’d vote for the bill. However, a recent opinion piece in The Hill by Devra Davis, titled “We Cannot Ignore the Dangers of Radiation in Our National Parks,” encourages people to oppose the bill because of “the damaging impacts of wireless radio frequency (RF) radiation — emitted by cellular installations — on all living creatures.” She concludes that “Expanding cell towers in parks without adequate safeguards will irrevocably harm wildlife, the environment and our encounters with the wild.”

Are Electromagnetic Fields
Making Me Ill?

The health risk of cell phone radiation is small to negligible. Russ Hobbie and I review much of the evidence of radio-frequency health effects in Section 9.10 of Intermediate Physics for Medicine and Biology. I also discuss this topic in my book Are Electromagnetic Fields Making Me Ill? In that publication, I specifically address Davis’s book Disconnect, which promotes a connection between cell phone radiation and cancer. My conclusions differ from hers. I wrote

Reviews such as these [for example, the FDA’s 2020 review] are a key reason the major health agencies do not believe that cell phones cause cancer. When agency scientists systematically weigh all the evidence, they consistently find no effect. The Centers for Disease Control and Prevention (CDC)—the US government federal agency that is responsible for protecting public health—is a bit more equivocal: “At this time we do not have the science to link health problems to cell phone use” [22]. The National Cancer Institute (NCI) is part of the US National Institutes of Health and is the primary federal agency for cancer research. Many of the nation’s best and brightest scientists and doctors work for, or are funded by, the NCI. Anyone who wants expert information about cancer should consult the NCI. On its website, it concludes that “the only consistently recognized biological effect of radiofrequency radiation absorption in humans that the general public might encounter is heating to the area of the body where a cell phone is held (e.g., the ear and head). However, that heating is not sufficient to measurably increase body temperature. There are no other clearly established dangerous health effects on the human body from radiofrequency radiation” [13].

Decide for yourself if you support the Connect Our Parks Act. I can see how cell phone reception could be vital for a hiker lost in the Grand Canyon but I don’t want people using their laptop to conduct a noisy zoom meeting in Yosemite. Do not, however, oppose the Connect Our Parks Act because of concerns about health hazards from electromagnetic radiation. There is little evidence that such hazards exist. If you want to examine the evidence yourself, get a copy of Are Electromagnetic Fields Making Me Ill? The Connect Our Parks Act is safe, but maybe not wise.

Philip Morse, Biological Physicist

This Sunday is the 120th anniversary of the birth of American physicist Philip Morse (1903–1985). Russ Hobbie and I mention Morse in Chapter 13 of our book Intermediate Physics for Medicine and Biology. We write

A classic textbook by Morse and Ingard (1968) provides a more thorough coverage of theoretical acoustics.

Theoretical Acoustics,
by Morse and Ingard.

The reference is to the book

Morse PM, Ingard KU (1968) Theoretical Acoustics. McGraw-Hill, New York.

In order to describe Morse’s life, I’ll quote excerpts from his obituary in the February, 1986 issue of Physics Today, written by his coauthor Herman Feshbach.

It was at Case [School of Applied Science, now Case Western Reserve University] that his lifelong interest in acoustics began. Morse received his BS in 1926, and pursued his graduate studies at Princeton University. It was a very exciting time, as the new quantum mechanics was the focus of attention.

Anyone who’s studied the vibrational states of molecules will probably have seen the Morse potential.

He wrote several papers alone and with Ernst Stueckelberg on molecular physics—in one of these he developed the “Morse potential.”

The Morse potential looks like the function plotted in Fig. 14.8 of IPMB, although we didn’t mention Morse by name in that chapter.

Morse joined MIT on the faculty. There he taught acoustics and quantum mechanics.

He gave advanced instruction to the brighter undergraduate students. One such undergraduate was Richard Feynman and the subject was quantum mechanics. At this time he renewed his interest in acoustics. A consequence was his book Vibration and Sound (1936), which he revised and expanded with Uno Ingard in 1968. Of equal importance to his book was his impact on the field: He brought up to date the methods employed by Lord Rayleigh and applied the results to practical problems of, for example, architectural acoustics.

Although Morse was not involved in the Manhattan Project, he did do applied physics research during World War II.

He and his colleagues played a decisive role in the defeat of the German submarine campaign. He gave a fascinating account of that effort in his autobiography, In At The Beginnings: A Physicist’s Life.

As influential as Morse’s book on acoustics is, his best-known book is probably the two-volume Methods of Theoretical Physics with Feshbach. That book is a little too advanced to be cited in IPMB, but I remember consulting it often during graduate school. 

 

Handbook of Mathematical Functions,
with Formulas, Graphs,
and Mathematical Tables.

Morse chaired the advisory committee that supervised the production of the Handbook of Mathematical Functions, with Formulas, Graphs, and Mathematical Tables.

Morse was the driving force behind the useful Handbook of Mathematical Functions, edited by Milton Abramovitz and Irene Stegun and produced by NBS [National Bureau of Standards] in 1964.

Feshbach concluded

Morse’s was truly a distinguished career, characterized by a unique breadth and fostered by his wide range of interests and his ability to initiate and develop new ventures. He was a dedicated scientist, or better, natural philosopher. As he wrote: “For those of us who like exploration, immersion in scientific research is not dehumanizing; in fact it is a lot of fun. And in the end, if one is willing to grasp the opportunities it can enable one to contribute something to human welfare.”

Would Morse have considered himself a biological physicist? Probably not. But his main interest was acoustics, and sound perception is inherently biological. In a few places Theoretical Acoustics deals with the physics of hearing. I’m comfortable declaring him an honorary biological physicist.

 

Happy birthday, Philip Morse!

John Moulder (1945–2022)

John Moulder,
from Khurana et al. (2008) Med. Phys.,
35:5203, with permission from Wiley.

John Moulder, a leading expert in radiation biology, died about a year ago (on July 17, 2022; I wasn’t aware of his death until last week). When Russ Hobbie and I discuss the possible health risks of weak electric and magnetic fields in Intermediate Physics for Medicine and Biology, we cite a website about powerlines and cancer “that unfortunately no longer exists.” (However, in a previous blog post I found that is does still exist.) We also cite several papers that Moulder wrote with his collaborator Ken Foster about potential electromagnetic field hazards, including

Moulder JE, Foster KR (1995) Biological Effects of Power-Frequency Fields as they Relate to Carcinogenesis. Proceedings of the Society for Experimental Biology and Medicine Volume 209, Pages 309–324.

Moulder JE, Foster KR (1999) Is There a Link Between Exposure to Power-Frequency Electric Fields and Cancer? IEEE Engineering in Medicine and Biology Magazine, Volume 18, Pages 109–116.

Moulder JE, Foster KR, Erdreich LS, McNamee JP (2005) Mobile Phones, Mobile Phone Base Stations and Cancer: A Review. International Journal of Radiation Biology, Volume 81, Pages 189–203.

Foster KR, Moulder JE (2013) Wi-Fi and Health: Review of Current Status and Research. Health Physics, Volume 105, Pages 561–575.

Perhaps my favorite of Moulder’s publications is his Point/Counterpoint article in the journal Medical Physics.

Khurana VG, Moulder JE, Orton CG (2008) There is Currently Enough Evidence and Technology Available to Warrant Taking Immediate Steps to Reduce Exposure of Consumers to Cell-Phone-Related Electromagnetic Radiation. Medical Physics, Volume 35, Pages 5203–5206.

Here is how Moulder is introduced in that paper.

Dr. Moulder obtained his Ph.D. in Biology in 1972 from Yale University. Since 1978, he has served on the faculty of the Medical College of Wisconsin, where he directs the NIH-funded Center for Medical Countermeasures Against Radiological Terrorism. His major research interests include the biological basis for carcinogenesis and cancer therapy, biological aspects of human exposure to non-ionizing radiation, and the prevention and treatment of radiation-induced normal tissue injuries. He has served on a number of national advisory groups concerned with environmental health, non-ionizing radiation, and radiological terrorism; and he currently serves as a radiation biology consultant to NASA.

Are Electromagnetic Fields
Making Me Ill?

In my book Are Electromagnetic Fields Making Me Ill? I wrote:

Radiation biologist John Moulder, of the Medical College of Wisconsin, began maintaining a website titled “Power Lines and Cancer FAQs [frequently asked questions],” which exhaustively summarized the evidence pro and con. Although this website is no longer available online, an archived pdf of it is [13]. In a 1996 article published by IEEE Engineering in Medicine and Biology, Moulder reviewed dozens of studies, and concluded that:

Given the relative weakness of the epidemiology, combined with the extensive and unsupportive laboratory studies, and the biophysical implausibility of interactions at relevant field strengths, it is often difficult to see why there is still any scientific controversy over the issue of power-frequency fields and cancer. [14]

13. large.stanford.edu/publications/crime/references/moulder/moulder.pdf. Access date: January 12, 2022. 

14. Moulder JE (1996) Biological Studies of Power-Frequency Fields and Carcinogenesis. IEEE Engineering in Medicine and Biology Magazine, Volume 15, Pages 31–49.

In a special issue of the International Journal of Radiation Biology dedicated to Moulder, Andrea DiCarlo and her colleagues discussed his work on radiological terrorism.

Through his awarded research grant and cooperative agreements from the NIH and beyond, John leaves behind a legacy of excellent, rigorous, and robust scientific findings, research collaborators who benefited from his expertise and dedication, and a cadre of well-trained students. Although it is impossible to list here all the lives that were touched, and the careers that were impacted by John’s influence, the authors can state with certainty that the field of medical preparedness for a radiation public health emergency would not be where it is now without the steadying hand and role played by Dr. Moulder, both in the early days in the program and during his final years as an active researcher. We are grateful for his years of research and join the entire radiation community in mourning the loss of a great investigator and person.

John Moulder, you were a voice of reason in a crazy world. We’ll miss you.

To hear Moulder in his own words, go to times 4:40 and 5:05 in this video about Power Line Fears.

https://www.youtube.com/watch?v=kf7KWkod3Zw

The Biological Physics Major

Today I want to talk to high school students who, when they attend college, might be majoring in biological physics. What does a biological physics major look like? Below I present my vision of a biological physics curriculum.

Foundational Courses

 Mathematics     

• Calculus 1 
• Calculus 2

Chemistry

• Chemistry 1 
• Chemistry 2 

Biology

• Biology 1 
• Biology 2 

Physics

• Physics 1 
• Physics 2
  

These are the core introductory courses that you absolutely must take. The physics class should be calculus-based. All the science classes need to have a laboratory component (whether as part of the introductory class or as a separate laboratory class to be taken concurrently with the lecture course). In a four-year undergraduate career, these classes represent one course per semester. You’ll probably take most of them in your freshman and sophomore years, because they’ll be prerequisites for more advanced courses. These foundational courses are required for just about any science or engineering major (including premed); if unsure what you want to study you can take them first and then decide your major once you know what you like best. 

Some high school students will have advanced placement credits for many of these classes, which is great, but it’s alright if you don’t. I’ll assume, however, that you’re ready to take calculus the first semester of your freshman year. The best thing you can do in high school to prepare for a biological physics major is to take enough math that you are ready for calculus on day one.

Advanced Courses

Mathematics 

• Multivariable Calculus 
• Differential Equations 

Chemistry 

• Organic Chemistry 
• Biochemistry 

Biology 

• Physiology 

Physics 

• Modern Physics 
• Thermodynamics 
• Electricity and Magnetism
  

Ideally you would take all these advanced courses, but there may be too many for that. Students coming in with advanced placement credits might be able to skip the introductory classes and take these instead. Some universities teach Biochemistry in the chemistry department, and some in the biology department. It doesn’t matter, take one. Many schools offer a two-semester sequence of Organic Chemistry. That would be okay, but it’s probably more Organic than you’ll need. In fact, if pressed for time you might skip Organic altogether, assuming you can still enroll in Biochemistry (which is essential) without needing Organic as a prerequisite. There are many biology classes you could add to this list, but I have included only the one I think is crucial: Physiology. 

If your school is like Oakland University, where I taught, you can take Modern Physics, which is basically a third semester of introductory physics with emphasis on modern topics (relativity, quantum mechanics, nuclear physics). If your school doesn’t have such a course, you could just take the physics department’s Quantum Mechanics class instead. I put E&M on my list because I think it’s the ultimate undergraduate physics course (and my favorite). I’ve noticed that other schools offering biological physics or biophysics curricula sometimes don’t include Electricity and Magnetism. I guess if you can’t cram all these advanced courses into four years and you have to skip something, consider skipping E&M.

Electives

Mathematics

• Linear Algebra 
• Probability and Statistics 

Chemistry

• Physical Chemistry 

Biology

• Genetics 
• Molecular Biology 
• Cell Biology 
• Anatomy 

Physics

• Nuclear Physics 
• Optics 
• Advanced Laboratory
  

I doubt you’ll have time for many of these electives. I believe your undergrad years are a time for getting a liberal education, so I would favor non-science electives over taking all these optional science courses. For example, be sure to take whatever classes are necessary to learn to write well. The Probability and Statistics course is higher priority than Linear Algebra. Don’t bother with Physical Chemistry unless you somehow got through all your physics courses without learning any quantum mechanics. I would load up on the elective biology classes only if you really love biology (you want to study BIOLOGICAL physics, not biological PHYSICS). The most important biology class in the list is, in my opinion, Anatomy. Nuclear Physics is useful if your interests lean toward medical physics; Optics if your interests tend toward biomedical engineering. If you plan on being an experimentalist, an Advanced Laboratory is valuable. 

For you readers who think I’m missing an essential class, or believe some of my recommendations are not needed, add your opinions to the comments section below. I’m sure readers would benefit from other points of view.

Specialty Courses

• Intermediate Physics for Medicine and Biology 1 
• Intermediate Physics for Medicine and Biology 2
  

I may have a vested interest here, but I think a two-semester sequence based on Intermediate Physics for Medicine and Biology should be mandatory. There are some topics in courses like these that are often not covered in other science classes, such as fluid dynamics, diffusion, feedback, and tomography. Not all universities offer such courses. In that case, you might have to take them via independent study, or just do some outside reading. Okay, if you must you can use some other textbook (but you’ll break my heart). 

Any faculty thinking of starting a biological physics major should note that these two specialty courses are the only classes that probably don’t already exist at your institution. The vast majority of the courses I list are already being taught at most colleges and universities.

Capstone Courses

• Colloquium 
• Independent Research
  

If your physics department has a colloquium course take it, at least during your senior year. If not, just attend regularly any research seminars hosted by your physics, chemistry, or biology departments (sometimes these events have free food!). I encourage you to do undergraduate research, even if it means you take fewer advanced courses and electives. My undergraduate research experience was more valuable than any class I ever took.  If your school has few research opportunities, search for a National Science Foundation-supported REU (Research Experience for Undergraduates).

Some students may be attending a college that has no biological physics or biophysics major. In that case, you will just have to adjust this list as best you can to fit your situation. Be flexible. If you have some oddball class that you love, or that is being taught by an outstanding and beloved instructor, don’t hesitate to substitute it for one of the courses on this list. Remember, it’s your education, so do as you want.

I hope this helps you high school students as you plan your college experience. Good luck!

A Short Course in Vector Calculus

Want a short course in vector calculus? You can find one in Intermediate Physics for Medicine and Biology.

Divergence

The divergence is defined in Section 4.1 in IPMB, when discussing the continuity equation. The divergence is one way to differentiate a vector field. I our case, the vector field is the current density (or some other type of flux density), j. Its divergence is defined as 

When you take the divergence of a vector (a quantity that has both magnitude and direction), you get a scalar (a quantity that has magnitude but no direction). In electrostatics, the electrical charge is conserved, implying that the divergence of the electrical current density is zero.

Curl

The curl is defined in Section 8.6, when analyzing electromagnetic induction. It is another way to differentiate a vector,

The symbols x̂, ŷ, and ẑ are unit vectors, and the vertical lines indicate that you follow the rules for determinants when expanding this expression. The curl appears often when analyzing the magnetic field. In our case, the curl of the electric field equations the negative of the time derivative of the magnetic field (Faraday’s law of induction).

Gradient

The gradient is a way to differentiate a scalar field to get a vector. 

 

You can think of the gradient, ∇, as representing the vector x̂ ∂/∂x + ŷ ∂/∂y + ẑ ∂/∂z. The divergence is then found by taking the dot product of the gradient with a vector, and the curl is found by taking the cross product of the gradient with the vector. In electrostatics, V represents of the electric potential (a scalar) and E represents the electric field (a vector). The two are related by

Laplacian

The Laplacian, ∇², is just the dot product of the gradient operator with itself. In other words 

 

You can apply the Laplacian to a vector, but it is more commonly applied to a scalar (such as electrical potential, temperature, or concentration). The Europeans use ∆ to represent the Laplacian, but that’s just weird and we Americans know better than that.

Other Coordinate Systems

We have written the divergence, curl, gradient, and Laplacian in Cartesian coordinates. These operators are more complicated in other coordinate systems. Appendix L of IPMB provides expressions for these operators in cylindrical coordinats of spherical coordinates.

The Divergence Theorem

The divergence theorem says that the volume integral of div J is equal to the surface integral of the normal component of J. We don’t dwell on this theorem in IPMB, but we do ask the reader to derive it in Homework Problem 4 of Chapter 4.

Stokes’ Theorem

We don’t discuss Stokes’ Theorem in IPMB, but I’ve pointed out how we might include a homework problem about it in a previous blog post. Stokes’ theorem says that the line integral of a vector around a closed loop is equal to the surface integral of the curl of that vector of an area bounded by the loop.

div, grad, curl, and all that,
by h. m. schey.

So, almost all the big concepts of vector calculus are presented in IPMB. If, however, you want a little more detail, Russ and I recommend the wonderful book div, grad, curl, and all that, by Harry Schey. I learned vector calculus from the first edition of that book as an undergraduate physics major at the University of Kansas. Schey died five years ago, but his book lives on.

Integral of the Bessel Function

Have you ever been reading a book, making good progress with everything making sense, and then you suddenly stop at say “wait… what?”. That happened to me recently as I was reading Homework Problem 31 in Chapter 12 of Intermediate Physics for Medicine and Biology. (Wait…what? I’m a coauthor of IPMB! How could there be any surprises for me?) The problem is about calculating the two-dimensional Fourier transform of 1/r, and it supplies the following Bessel function identity 

The function J₀ is a Bessel function of the first kind of order zero. What surprised me is that if you let x = kr, you get that the integral of the Bessel function is one,

Really? Here’s a plot of J₀(x).

It oscillates like crazy and the envelope of those oscillations falls off very slowly. In fact, an asymptotic expansion for J₀ at large x is

The leading factor of 1/√x decays so slowly that its integral from zero to infinity does not converge. Yet, when you include the cosine so the function oscillates, the integral does converge. Here’s a plot of

The integral approaches one at large x, but very slowly. So, the expression given in the problem is correct, but I sure wouldn’t want to do any numerical calculations using it, where I had to truncate the endpoint of the integral to something less than infinity. That would be a mess!

Here’s another interesting fact. Bessel functions come in many orders—J₀, J₁, J₂, etc.—and they all integrate to one.

Who’s responsible for these strangely-behaved functions? They’re named after the German astronomer Friedrich Bessel but they were first defined by the Swiss mathematician Daniel Bernoulli (1700–1782), a member of the brilliant Bernoulli family. The Bernoulli equation, mentioned in Chapter 1 of IPMB, is also named for Daniel Bernoulli. 

There was a time when I was in graduate school that I was obsessed with Bessel functions, especially modified Bessel functions that don’t oscillate. I’m not so preoccupied by them now, but they remain my favorite of the many special functions encountered in physics.